Answered step by step
Verified Expert Solution
Question
1 Approved Answer
2. Let n E N. Suppose n chords are drawn in a circle in such a way that each chord intersects every other, but
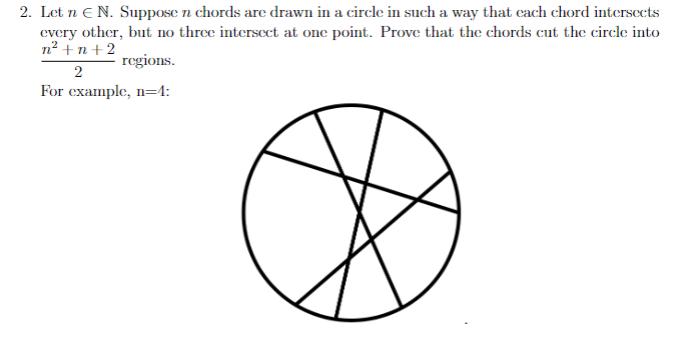
2. Let n E N. Suppose n chords are drawn in a circle in such a way that each chord intersects every other, but no three intersect at one point. Prove that the chords cut the circle into n +n +2 regions. 2 For example, n=1:
Step by Step Solution
There are 3 Steps involved in it
Step: 1
Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2
Step: 3
Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started
Financial Algebra advanced algebra with financial applications
Authors: Robert K. Gerver
1st edition
978-1285444857, 128544485X, 978-0357229101, 035722910X, 978-0538449670
