Answered step by step
Verified Expert Solution
Question
1 Approved Answer
2. Let X1, X2,...,^ be independent and identically distributed random variables from a Pareto distribution with probability density function given by Px(x|0) = 0
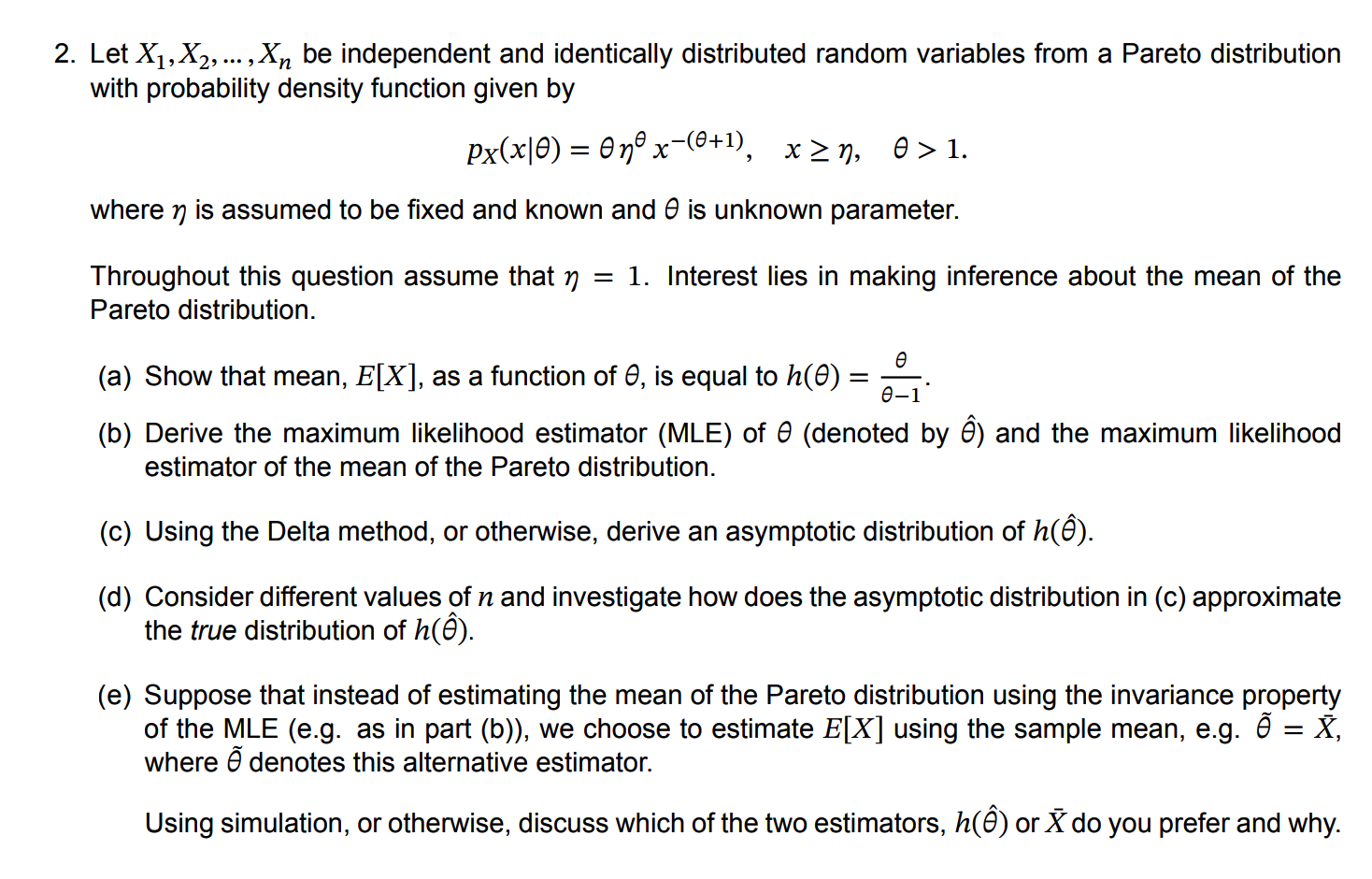
2. Let X1, X2,...,^ be independent and identically distributed random variables from a Pareto distribution with probability density function given by Px(x|0) = 0 x(0+1), x = n, 0 > 1. where n is assumed to be fixed and known and O is unknown parameter. Throughout this question assume that n = 1. Interest lies in making inference about the mean of the Pareto distribution. (a) Show that mean, E[X], as a function of e, is equal to h(e): = 0-1 (b) Derive the maximum likelihood estimator (MLE) of (denoted by ) and the maximum likelihood estimator of the mean of the Pareto distribution. (c) Using the Delta method, or otherwise, derive an asymptotic distribution of h(). (d) Consider different values of n and investigate how does the asymptotic distribution in (c) approximate the true distribution of h(). (e) Suppose that instead of estimating the mean of the Pareto distribution using the invariance property of the MLE (e.g. as in part (b)), we choose to estimate E[X] using the sample mean, e.g. 0 = X, where denotes this alternative estimator. Using simulation, or otherwise, discuss which of the two estimators, h() or X do you prefer and why.
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


