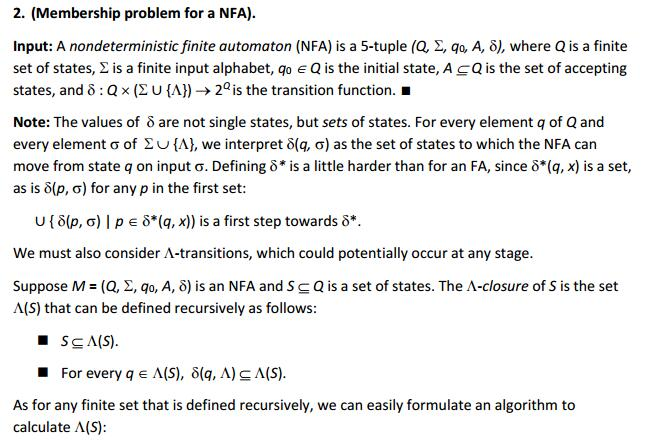
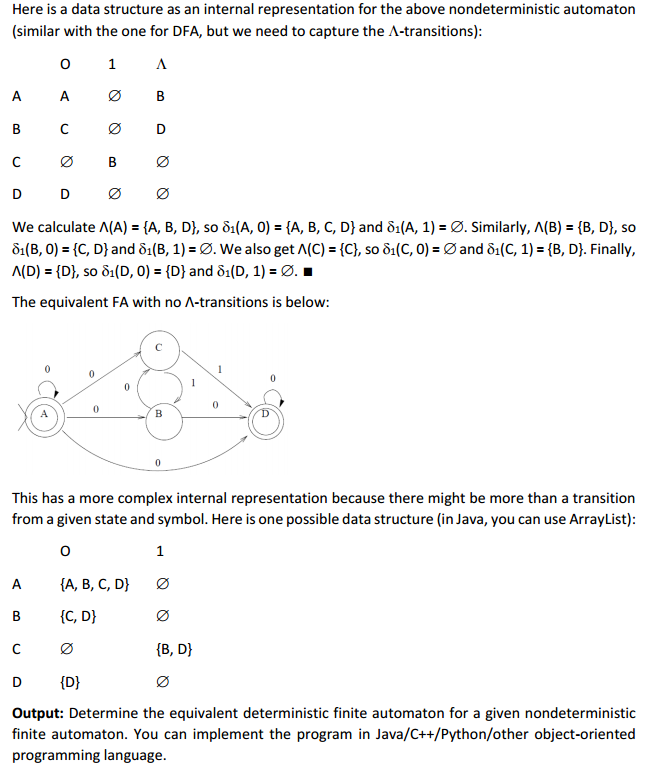
2. (Membership problem for a NFA). Input: A nondeterministic finite automaton (NFA) is a 5-tuple (Q, Y, qa, A, o), where Q is a finite set of states, is a finite input alphabet, qo e Q is the initial state, A CQ is the set of accepting states, and Q x U (A) 2 is the transition function. Note: The values of are not single states, but sets of states. For every element q of Q and every element o of {A), we interpret 5(q, G) as the set of states to which the NFA can move from state q on input o. Defining is a little harder than for an FA, since (q, x) is a set, as is o(p, o) for any p in the first set: u{6(p, o) l p E (q, x)) is a first step towards o*. We must also consider A-transitions, which could potentially occur at any stage. Suppose M- (Q, E, go, A, o) is an NFA and Sg Qis a set of states. The A-closure of S is the set A(S) that can be defined recursively as follows: Sg (S) For every q E A(S), (q, A) cA(S). As for any finite set that is defined recursively, we can easily formulate an algorithm to calculate A(S): 2. (Membership problem for a NFA). Input: A nondeterministic finite automaton (NFA) is a 5-tuple (Q, Y, qa, A, o), where Q is a finite set of states, is a finite input alphabet, qo e Q is the initial state, A CQ is the set of accepting states, and Q x U (A) 2 is the transition function. Note: The values of are not single states, but sets of states. For every element q of Q and every element o of {A), we interpret 5(q, G) as the set of states to which the NFA can move from state q on input o. Defining is a little harder than for an FA, since (q, x) is a set, as is o(p, o) for any p in the first set: u{6(p, o) l p E (q, x)) is a first step towards o*. We must also consider A-transitions, which could potentially occur at any stage. Suppose M- (Q, E, go, A, o) is an NFA and Sg Qis a set of states. The A-closure of S is the set A(S) that can be defined recursively as follows: Sg (S) For every q E A(S), (q, A) cA(S). As for any finite set that is defined recursively, we can easily formulate an algorithm to calculate A(S)