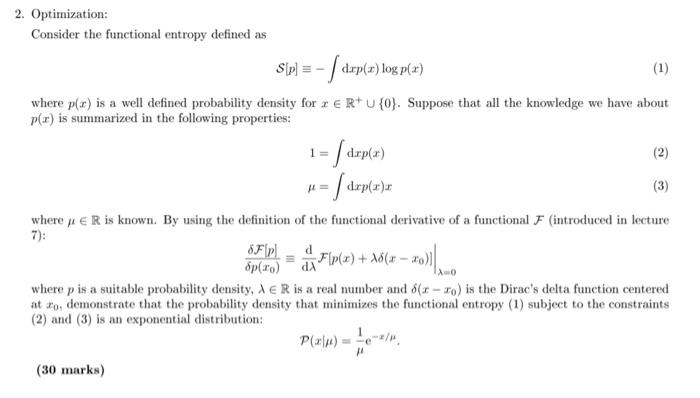
2. Optimization: Consider the functional entropy defined as S[p] = -dap(z) log p(x) (1) where p(x) is a well defined probability density for a ERU {0}. Suppose that all the knowledge we have about p(x) is summarized in the following properties: 1= [drp(2 = [dap(x)x (2) (3) where ER is known. By using the definition of the functional derivative of a functional F (introduced in lecture 7): (30 marks) SF p =F\p(x) + A8(x - p(ro) where p is a suitable probability density, AER is a real number and 8(x-ro) is the Dirac's delta function centered at zo, demonstrate that the probability density that minimizes the functional entropy (1) subject to the constraints (2) and (3) is an exponential distribution: P(x)= - FO)1|AMO "1