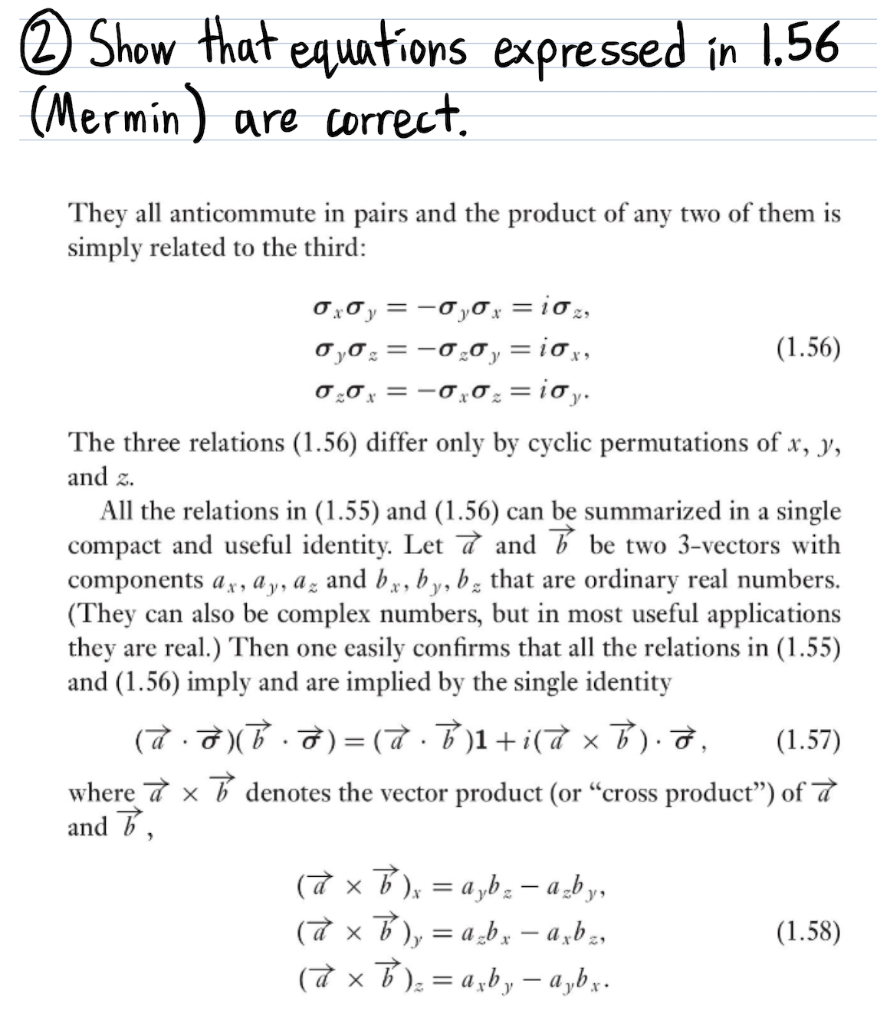
2 Show that equations expressed in 1.56 (Mermin) are correct. They all anticommute in pairs and the product of any two of them is simply related to the third: == = (1.56) The three relations (1.56) differ only by cyclic permutations of x, y, and z. All the relations in (1.55) and (1.56) can be summarized in a single compact and useful identity. Let a and b be two 3-vectors with components ax, ay, az and bx, by, bg that are ordinary real numbers. (They can also be complex numbers, but in most useful applications they are real.) Then one easily confirms that all the relations in (1.55) and (1.56) imply and are implied by the single identity . = . X . (1.57) where denotes the vector product (or "cross product") of and b, ( b ) = a,b a zby, (a x) =ababa ( b ) = aby aybx. (1.58)