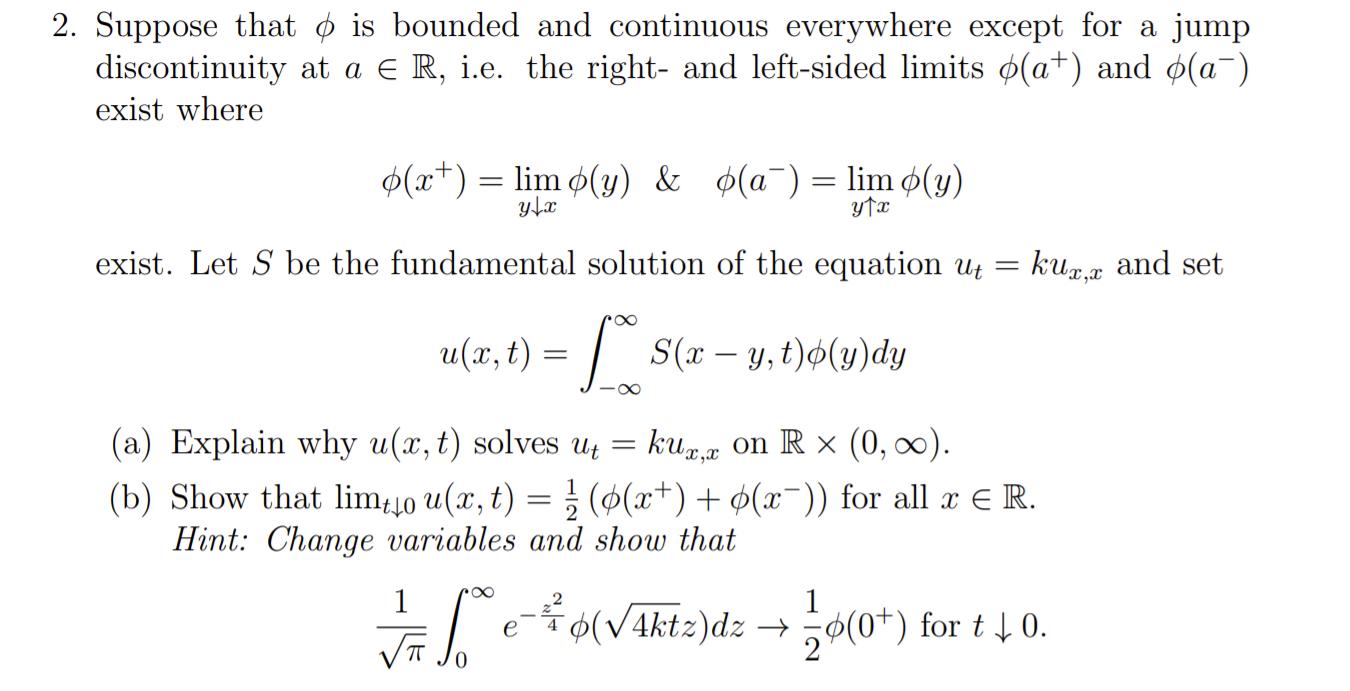
2. Suppose that o is bounded and continuous everywhere except for a jump discontinuity at a e R, i.e. the right- and left-sided limits (a+) and (a-) exist where $(x*) = lim (y) & (a) = lim o(y) ytx exist. Let S be the fundamental solution of the equation u = kuza and set u(x,t) : | S(x y,t)(y)dy || (a) Explain why u(x, t) solves U = kua on R (0, 0). (b) Show that lim40 u(x, t) = } ($(x+)+(x)) for all a E R. Hint: Change variables and show that 1 $(V4ktz)dz (0*) for t 4 0. e