Question
2. Suppose the number of steps required in the worst case for two algorithms are as follows: Algorithm 1: f(n)= 3n + 5 Algorithm
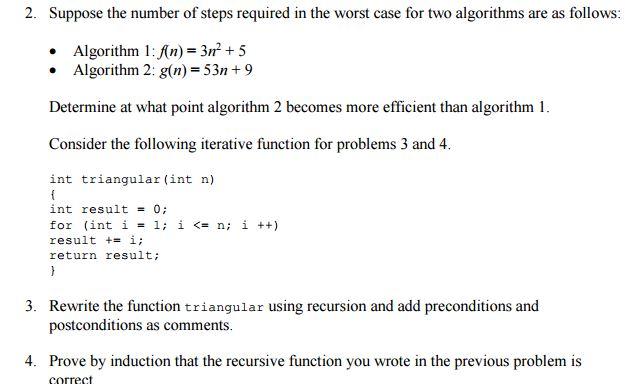
2. Suppose the number of steps required in the worst case for two algorithms are as follows: Algorithm 1: f(n)= 3n + 5 Algorithm 2: g(n) = 53n +9 Determine at what point algorithm 2 becomes more efficient than algorithm 1. Consider the following iterative function for problems 3 and 4. int triangular (int n) { int result = 0; for (int i = 1; i
Step by Step Solution
There are 3 Steps involved in it
Step: 1
A nice problem in computational complexity and recursion Problem 2 To determine at what point algori...
Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get StartedRecommended Textbook for
Introduction to Data Mining
Authors: Pang Ning Tan, Michael Steinbach, Vipin Kumar
1st edition
321321367, 978-0321321367
Students also viewed these Programming questions
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
View Answer in SolutionInn App



