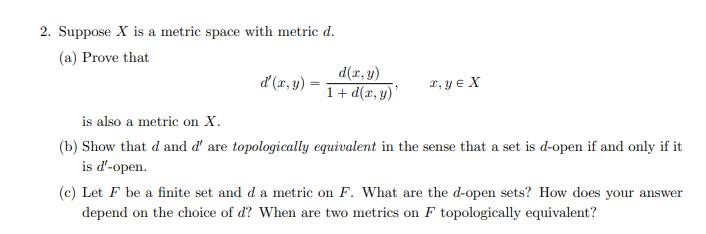
2. Suppose X is a metric space with metric d. (a) Prove that d(r, y) 1+ d(x, y)' d'(r, y) 2, y e X is also a metric on X. (b) Show that d and d' are topologically equivalent in the sense that a set is d-open if and only if it is d'-open. (c) Let F be a finite set and d a metric on F. What are the d-open sets? How does your answer depend on the choice of d? When are two metrics on F topologically equivalent?