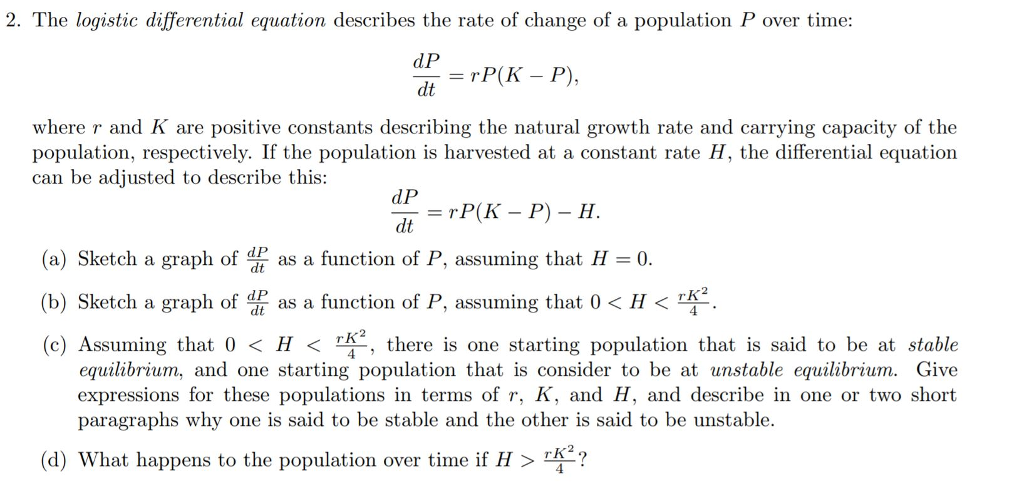
2. The logistic differential equation describes the rate of change of a population P over time: dP dt =rP(K-P), where r and K are positive constants describing the natural growth rate and carrying capacity of the population, respectively. If the population is harvested at a constant rate H, the differential equation can be adjusted to describe this: dP dt =rP(KP) - H. (a) Sketch a graph of d dP as a function of P, assuming that H = 0. (b) Sketch a graph of d as a function of P, assuming that 0