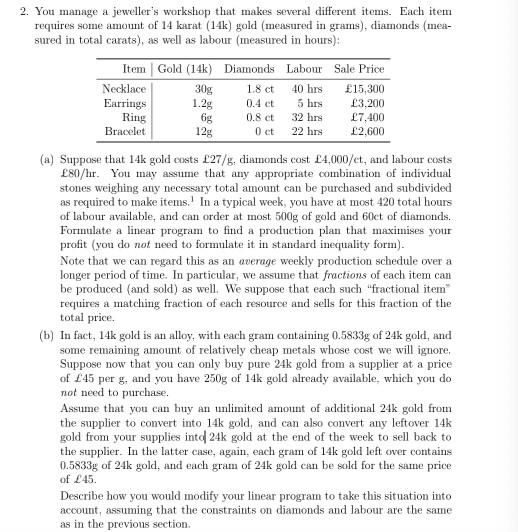
2. You manage a jeweller's workshop that makes several different items. Each item. requires some amount of 14 karat (14k) gold (measured in grams), diamonds (mea- sured in total carats), as well as labour (measured in hours): Item Gold (14k) Diamonds Labour Sale Price Necklace 30g 1.8ct 40 hrs 15,300 Earrings 1.2g 0.4 ct 5 hrs 3,200 Ring 6g 0.8 ct 32 hrs 7,400 Bracelet 12g 0 ct 22 hrs 2,600 (a) Suppose that 14k gold costs 27/g. diamonds cost 4,000/et, and labour costs 80/hr. You may assume that any appropriate combination of individual stones weighing any necessary total amount can be purchased and subdivided as required to make items. In a typical week, you have at most 420 total hours of labour available, and can order at most 500g of gold and 60ct of diamonds. Formulate a linear program to find a production plan that maximises your profit (you do not need to formulate it in standard inequality form). Note that we can regard this as an average weekly production schedule over a longer period of time. In particular, we assume that fractions of each item can be produced (and sold) as well. We suppose that each such "fractional item" requires a matching fraction of each resource and sells for this fraction of the total price. (b) In fact, 14k gold is an alloy, with each gram containing 0.5833g of 24k gold, and some remaining amount of relatively cheap metals whose cost we will ignore. Suppose now that you can only buy pure 24k gold from a supplier at a price of 45 per g, and you have 250g of 14k gold already available, which you do not need to purchase. Assume that you can buy an unlimited amount of additional 24k gold from the supplier to convert into 14k gold, and can also convert any leftover 14k gold from your supplies into 24k gold at the end of the week to sell back to the supplier. In the latter case, again, each gram of 14k gold left over contains 0.5833g of 24k gold, and each gram of 24k gold can be sold for the same price of 45. Describe how you would modify your linear program to take this situation into account, assuming that the constraints on diamonds and labour are the same as in the previous section.