Question: 20. Given: BC = CD, AB=BD Prove: ABCD A B C D First, show that BC = CD and BC + CD = BD.

20. Given: BC = CD, AB=BD Prove: ABCD A B C D

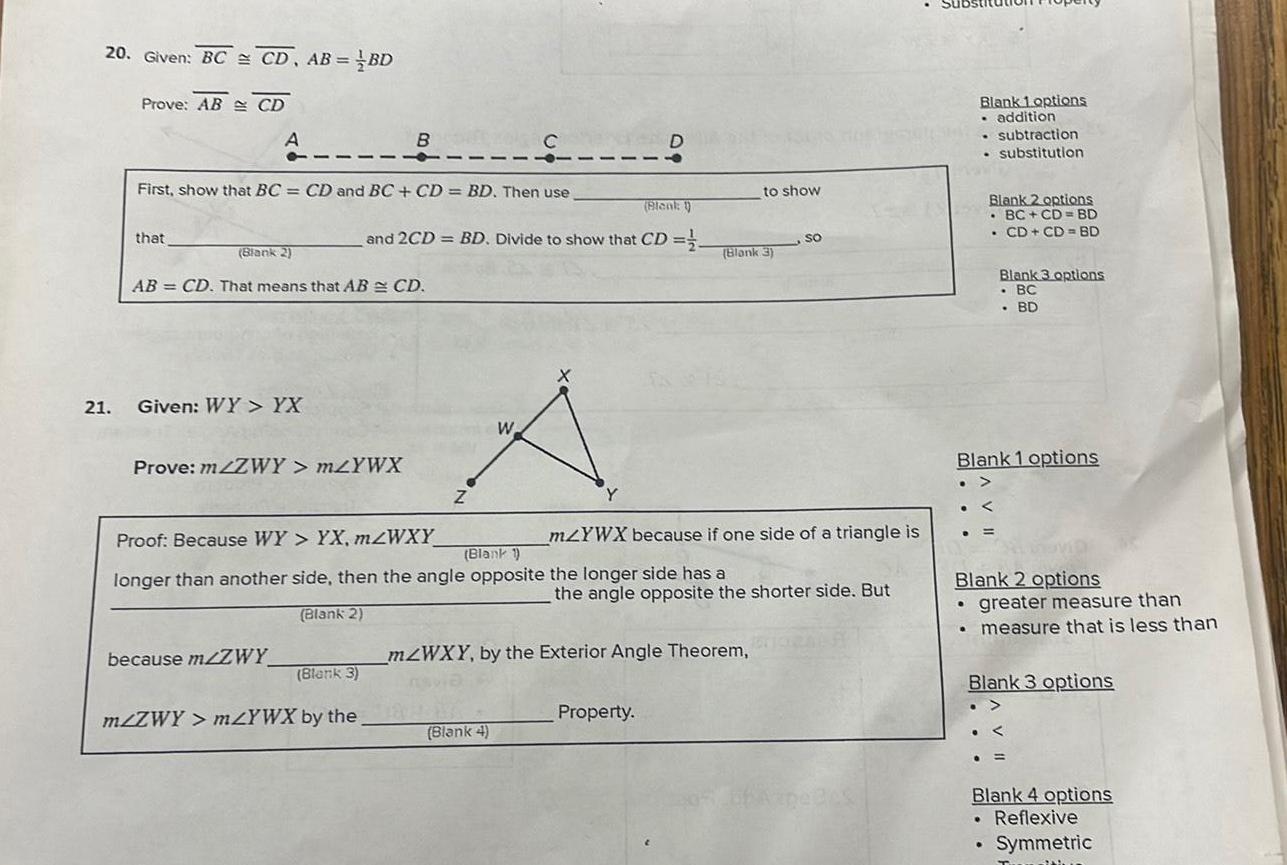
20. Given: BC = CD, AB=BD Prove: ABCD A B C D First, show that BC = CD and BC + CD = BD. Then use to show (Blank) that and 2CD = BD. Divide to show that CD= SO (Blank 2) (Blank 3) ABCD. That means that ABCD. 21. Given: WY > YX W Blank 1 options addition subtraction substitution Blank 2. options .BC+CD BD CD+CD=BD Blank 3 options . BC . BD Prove: mZZWY > mzYWX Z (Blank 1) Y mZYWX because if one side of a triangle is Proof: Because WY > YX, mZWXY longer than another side, then the angle opposite the longer side has a the angle opposite the shorter side. But (Blank 2) because mZZWY mZWXY, by the Exterior Angle Theorem, (Blank 3) mZZWY > mZYWX by the Property. (Blank 4) Blank 1 options . > < Blank 2 options greater measure than measure that is less than Blank 3 options Blank 4 options Reflexive Symmetric

Step by Step Solution

There are 3 Steps involved in it

1 Expert Approved Answer

Step: 1 Unlock blur-text-image

Question Has Been Solved by an Expert!

Get step-by-step solutions from verified subject matter experts

Step: 2 Unlock

Step: 3 Unlock

Students Have Also Explored These Related Mathematics Questions!

...Thanks. 14-36. + Consider the data from the first replicate of Track cross-country skier (10 or 20 min), and delay between Exercise 14-14. the time of juice intake (0 or 20 min) and the beginning...

Using the threshold support of two observations for the following table Transactional Database Items 1 ALON A B C D E, D, C, A, E B. D. A D. C, BA F. D.GE 5 FIND THE SECOND LEVEL ITEMSET? Choose FIND...

DIRECTIONS: For each of the following questions, you may only site propositions 6.1, 7.1, 7.2, and 7.3; the incidence axioms, the betweenness axioms, and any definition from sections 6 & 7 of...

ST332 & ST409 Medical Statistics 2014-15: Exercises 2 1) Clinical Trial Design (based on an old exam question) A new drug against AIDS has been developed that may be useful in conjunction with the...

Using the threshold support of two observations for the following table Transactional Database Items TID 11 AONI A, B, C, D E, D, C, A, E B. D. A D. C, BA F. D. GF 5 FIND THE SECOND LEVEL ITEMSET?...

hi I need help with my chapter 7 payroll project 2021 edition , i would really appreciate your help Start of Payroll Project Project Audit Test ? Net Paid $12,340.13 i am trying to attach the excell...

S.Y. 2021-2022 FIRST NAME M.I. LAST NAME am imsona Rogen LIT Parent's Signature: Grade & Section: Date: Score: Directions: Shade the letter of the correct answer. Show all necessary SOLUTIONS in a...

Show all the work, step by step, please: Encrypt the first 4 letters of your last name as a block of data to be encrypted. (Let A=1, B=2, C=3,Z=26). Decrypt the encrypted number to demonstrate that...

these are some geometry worksheets. if you have any questions please let me know!!!!! \fProperties of Triangles Circuit Directions: Begin with problem #1. Perform the operation(s) necessary to arrive...

\"Lady Tasting Tea\"1 R. A. Fisher was one of the founding fathers of modern statistics. One of his early, and perhaps the most famous, experiments was to test an English lady's claim that she could...

Two countries have the same effectiveness of labor production function: Y = F (K.LE) = K^0.5 (LE)^0.5 a. what is the per effective worker production function for these countries? b. what is the...

If one force of 5kN co-axial with element 3 is applied at joint 2, and the other force of 4kN co-axial with element 5 is applied at joint 3, write the net stiffness equations by introducing the nodes...

Land was acquired for a future building site at a cost of $ 4 1 , 5 0 0 . The assessed valuation for tax purposes is $ 2 7 , 0 0 0 , a qualified appraiser placed its value at $ 4 9 , 0 0 0 , and a...

Design a circular positional list ADT that abstracts a circularly linked list in the same way that the positional list ADT abstracts a doubly linked list.

In each of the following settings, decide whether you should use two sample t procedures to perform inference about a difference in means or paired t procedures to perform inference about a mean...

Using the Laplace transform pairs of Table 2.1 and the Laplace transform theorems of Table 2.2, derive the Laplace transforms for the following time functions: a. e -at sin t u(t) b. e -at cos t u(t)...

A gas is dissolved in a liquid. When the temperature of the solution is increased, the solubility of the gas a. increases b. decreases c. remains the same d. any of these, depending upon the nature...

On December 11, 1998, the Mars Climate Orbiter was launched on a 760 million mile journey to the Red Planet. On September 23, 1999, a final rocket firing was to put the spacecraft into orbit, but it...

Tori and some other childeen experimented with balls to see which ones rolled. First using a piece of cardboard to use as a ramp, they angled it on a block to the floor to test the balls. Then Tori...

(Worksheet Analysis of Selected Accounts) Data for Anita Baker Company are presented in E23-18. Instructions Prepare entries in journal form for all adjustments that should be made on a worksheet for...

(Worksheet Analysis of Selected Transactions) The transactions below took place during the year 2008. Instructions Show by journal entries the adjustments that would be made on a worksheet for a...

A manager in your organization just received a special order at a price that is "below cost." The manager points to the document and says: "These are the kinds of orders that will get you in trouble....