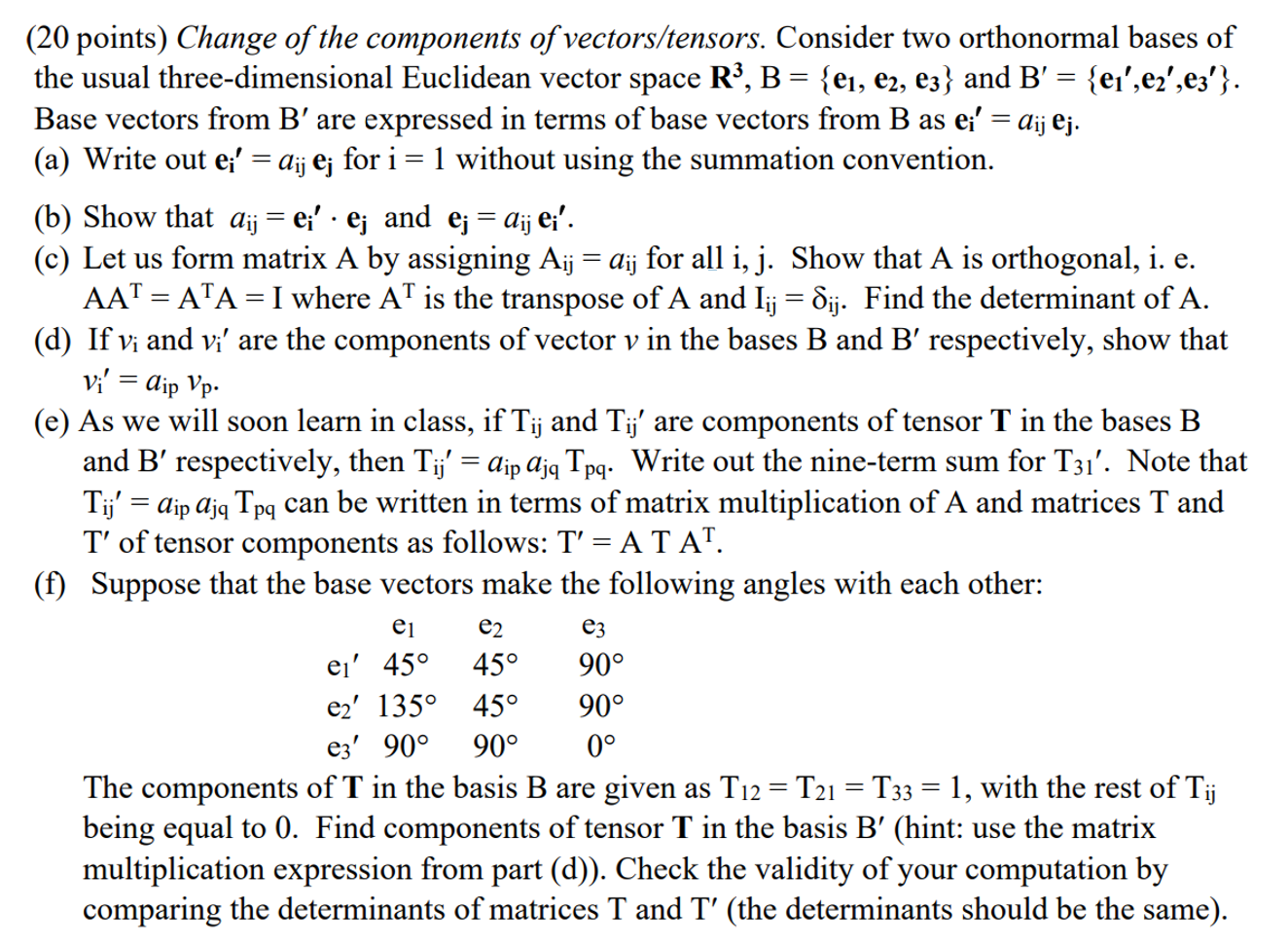
(20 points) Change of the components of vectors/tensors. Consider two orthonormal bases of the usual three-dimensional Euclidean vector space R, B = {e1, e2, e3} and B' = {e1',e2',e3'}. Base vectors from B' are expressed in terms of base vectors from B as ei' = aij ej. (a) Write out e' = ij e for i = 1 without using the summation convention. (b) Show that dij = e' ej and e = aij ei'. (c) Let us form matrix A by assigning Aij = ij for all i, j. Show that A is orthogonal, i. e. AAT = ATA = I where A is the transpose of A and Ij = Sij. Find the determinant of A. (d) If vi and vi' are the components of vector v in the bases B and B' respectively, show that Vi=aip Vp. (e) As we will soon learn in class, if Tij and Tij' are components of tensor T in the bases B and B' respectively, then Tij' = ip jq Tpq. Write out the nine-term sum for T31'. Note that Tij'aip aja Tpq can be written in terms of matrix multiplication of A and matrices T and T' of tensor components as follows: T' = ATAT. (f) Suppose that the base vectors make the following angles with each other: e1 e2 e1' 45 45 90 e2' 135 45 90 e3' 90 90 0 The components of T in the basis B are given as T12 = T21 = T33 = 1, with the rest of Tij being equal to 0. Find components of tensor T in the basis B' (hint: use the matrix multiplication expression from part (d)). Check the validity of your computation by comparing the determinants of matrices T and T' (the determinants should be the same).