Question: 20 points Let X be a nonnegative integer valued random variable (i.e., X = {0, 1, 2, . . .}). We will prove that:

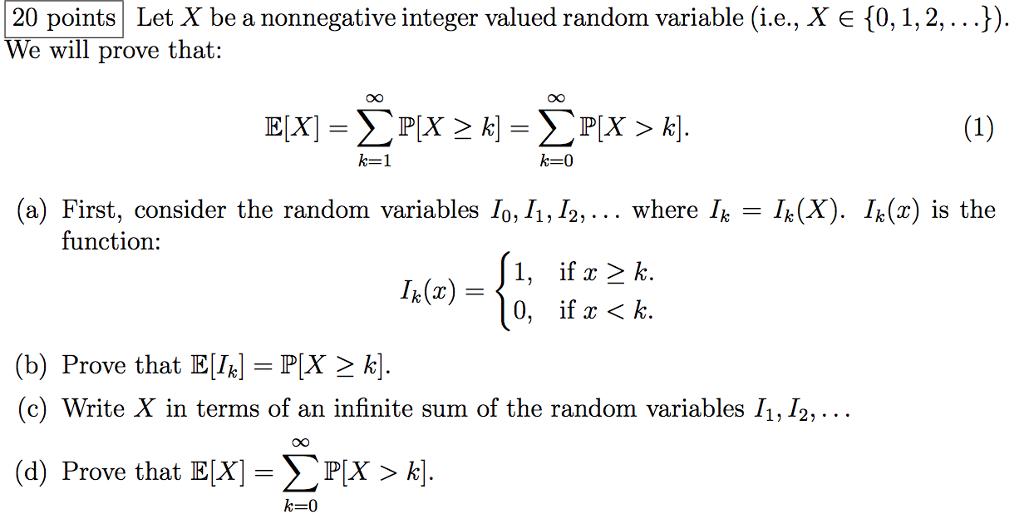
20 points Let X be a nonnegative integer valued random variable (i.e.,

20 points Let X be a nonnegative integer valued random variable (i.e., X = {0, 1, 2, . . .}). We will prove that: E[X] = P[X > k] = P[X > k]. k=1 k=0 (1) (a) First, consider the random variables I0, 11, 12,... where Ik = Ik(X). Ik(x) is the function: (1, if x > k. Ik(x) = if x < k. (b) Prove that E[Ik] = P[X > k]. (c) Write X in terms of an infinite sum of the random variables I, 12, ... (d) Prove that E[X] = P[X > k]. k=0

Step by Step Solution

There are 3 Steps involved in it

1 Expert Approved Answer

Step: 1 Unlock blur-text-image

Question Has Been Solved by an Expert!

Get step-by-step solutions from verified subject matter experts

Step: 2 Unlock

Step: 3 Unlock

Students Have Also Explored These Related Finance Questions!

The 5-kg spring-loaded gun rests on the smooth surface. It fires a ball having a mass of 1 kg with a velocity of v' = 6 m/s relative to the gun in the direction shown. If the gun is originally at...

Mr. Jacobs has a both a pension and a 401k at his company. His pension will provide a $3,000 a month benefit upon his retirement and his 401k currently has a $325,000 balance. Additionally, his...

Delauder Enterprises makes a variety of products that it sells to other businesses. The company's activity-based costing system has four activity cost pools for assigning costs to products and...

Comparative financial statements for Heritage Antiquing Services for the fiscal year ending December 31 appear on the following page. The company did not issue any new common or preferred stock...

14. The Discrete Hazard Rate Method: Let X denote a nonnegative integer valued random variable. The function (n) = P{X = n | X n}, n 0, is called the discrete hazard rate function. (a) Show that P{X...

14. The Discrete Hazard Rate Method: Let X denote a nonnegative integer valued random variable. The function () = P[X = n\X> n], > 0, is called the discrete hazard rate function. (a) Show that P{X =...

The Discrete Hazard Rate Method: Let X denote a nonnegative integer valued random variable. The function (n) = P{X = n|X n},n 0, is called the discrete hazard rate function. (a) Show that P{X = n}...

probability question Q3 (a) Let X be a nonnegative integer valued random variable with probability mass function px(k). Derive an expression for P(X is odd) in terms of its probability generating...

Let X be a nonnegative integer valued random variable with a pdf fx (x) satisfying the relation fx (a) = 4fx (2 - 1) x = 1, 2, 3, ... (a) Explicitly evaluate the pdf f. (b) Factorial Moment...

46. If X is a nonnegative integer valued random variable, show that Hint: Define the sequence of random variables In, n 1, by Now express X in terms of the In. (b) If X and Y are both nonnegative...

Prepare asientos de diario para registrar las siguientes transacciones comerciales de Lowe's, que utiliza el sistema de inventario perpetuo y el mtodo bruto. (Sugerencia: ayudar a identificar cada...

28. Yakuza Company issued 2,500 share of P25 par value preference shares with detachable warrants. The security package sells for P105. Each warrant enables the holder to purchase two shares of P10...

Good Vibes Manufacturing is a firm with an annual net income of $20 million, revenue of $60 million and cost of goods sold of $25 million. If the balance sheet amounts show $2.5 million of inventory...

Review and discuss the collapse of the Futures Oil Market, which fell into the negative realm in May 2020. What were the main reasons for this fall into the negative realm? Critically discuss. (20...

Alfie Lockyer decides to start up a business as a sole trader on 1 July 2020. Alfies Rugby League Memorabilia and Framing Services will sell a range of different memorabilia items including framed...

A video conference application wants to keep the end-to-end packet delay below 200ms, and at a rate of at least 1Mb/s. The packets are all 10,000bits long and pass through 9 routers (and therefore...

The distance travelled (in km) by a courier service motorcycle on 50 trips was recorded by the driver. 24 30 20 28 23 17 16 21 20 18 19 22 26 31 21 13 15 20 18 20 34 29 24 23 25 17 35 29 19 10 17 11...

True or false: Typically only individuals at the lower levels of an organization present obstacles to change. True False

Complete the coverage probability calculation needed in Example 9.2.17. a. If X22Y is a chi squared random variable with Y ~ Poisson(A), show that E(x22y) = 2Î», Var(x22Y) =...

Prove the identity for the negative binomial distribution given in Theorem 3.6.8, part (b).

Suppose that the random variable Y has a binomial distribution with n trials and success probability X, where n is a given constant and X is a uniform(0,1) random variable. (a) Find EE and Var Y. (b)...

A petty cash fund is established at $100. At the end of May, the fund has a cash balance of $36 and petty cash vouchers for postage, $29, and office supplies, $34. Prepare the journal entry on May...

On June 30, the end of the current fiscal year, the following information is available to BND Corporation's accountants for making adjusting entries: a. Among the liabilities of the company is a...

The accounting records of Braintree Foods, Inc., include the following items at December 31, 2017: Requirements 1. Show how each relevant item would be reported on the Braintree Foods, Inc.,...