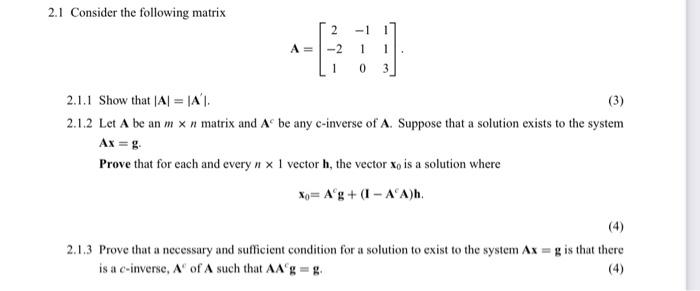
2.1 Consider the following matrix 2 -61 0 A = 2.1.1 Show that |A| = |A'|. (3) 2.1.2 Let A be an m x n matrix and A be any c-inverse of A. Suppose that a solution exists to the system Ax = g. Prove that for each and every x 1 vector h, the vector xo is a solution where Xo Ag+ (1-AA)h. 2.1.3 Prove that a necessary and sufficient condition for a solution to exist to the system Ax = g is that there is a c-inverse, A of A such that AA'g=g. (4)