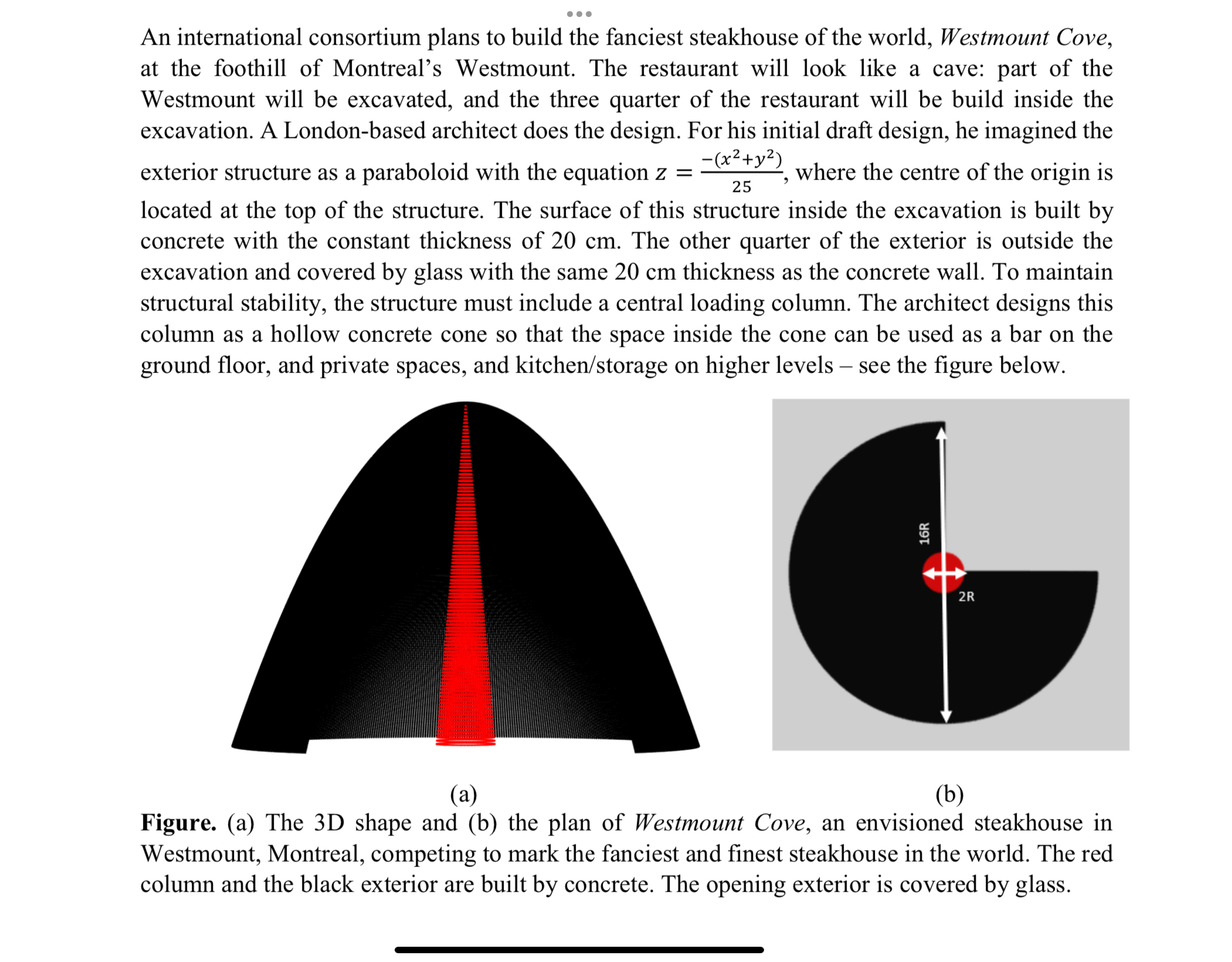
, 25 An international consortium plans to build the fanciest steakhouse of the world, Westmount Cove, at the foothill of Montreal's Westmount. The restaurant will look like a cave: part of the Westmount will be excavated, and the three quarter of the restaurant will be build inside the excavation. A London-based architect does the design. For his initial draft design, he imagined the exterior structure as a paraboloid with the equation z = -(x+y), where the centre of the origin is located at the top of the structure. The surface of this structure inside the excavation is built by concrete with the constant thickness of 20 cm. The other quarter of the exterior is outside the excavation and covered by glass with the same 20 cm thickness as the concrete wall. To maintain structural stability, the structure must include a central loading column. The architect designs this column as a hollow concrete cone so that the space inside the cone can be used as a bar on the ground floor, and private spaces, and kitchen/storage on higher levels see the figure below. - 16R 2R (a) (b) Figure. (a) The 3D shape and (b) the plan of Westmount Cove, an envisioned steakhouse in Westmount, Montreal, competing to mark the fanciest and finest steakhouse in the world. The red column and the black exterior are built by concrete. The opening exterior is covered by glass. To make the structure safe from design standpoint, the structural engineer from Montreal determines that the radius of the hollow cone column must be at least one eighth of the radius for the base for paraboloid and its wall thickness should be at least 35 cm. The crowns of the cone and the paraboloid meet the same point, which is the top of the structure. Assuming again this point as the centre of the origin, the architect used z = -25.6 x + y as the equation for the column. While the consortium's board likes the design, they have a major hesitation regarding the cost of constructing such a magnificent design. They agreed in principle with the design but want to cap the structural cost for constructing the concrete and glass walls and the loading column to $2M at the very most. This cost should include the price of the concrete for the exterior walls inside the excavation as well as the hollow cone column, along with the price of the glass wall. The price of concrete is $500/m and includes the construction labor and transportation costs too. The unit price for exterior glass wall can be calculated by $500 times the unit area multiplied by its thickness, multiplied by its curvature plus a fixed $500 for each meter of height. The only supplier of a such a glass wall is an Italian manufacturer based in Milan that uses the formula of = |d d d 2 23/2 dx2 dy2 dxdy 3/2 to calculate the curvature of its glass production. The quoted price of glass wall (1+ (az) + (af) ) * dx also includes labor work, transportation, and installation cost. Using the theory of multiple integral and your general knowledge of calculus as a whole: a) Find the price of all concrete works including three quarter of outer paraboloid and the whole inner cone as a function of radius of paraboloid R*; R* = 8R (30%). b) Find the price of glass wall as a function of R* (30%). c) What would be the critical value of R* after which the price exceeds $2M. Is this critical design feasible? Look at the feasibility of design from the perspective of the guest space (1 + (a2) + (az)) also includes labor work, transportation, and installation cost. Using the theory of multiple integral and your general knowledge of calculus as a whole: a) Find the price of all concrete works including three quarter of outer paraboloid and the whole inner cone as a function of radius of paraboloid R*; R* = 8R (30%). b) Find the price of glass wall as a function of R*(30%). c) What would be the critical value of R* after which the price exceeds $2M. Is this critical design feasible? Look at the feasibility of design from the perspective of the guest space (i.e., three quarter of the base of paraboloid minus the base area of the cone) and the height of the celling. Do you think that the architect can convince the board that his design will be certainly cost less than $2M (30%)? d) Before taking the design to the board, the architect needs to finalize the shape of the cone and paraboloid. Imagine the general equation of cone as z = -ax + y and for the paraboloid as z = -(x+y). Given the fact the radius of cone is one eighth of the radius of b the paraboloid, find b as a function of R* and a, knowing that the top of the cone and paraboloid should meet at their very top (10%). 1 The structural engineer is a Concordia alumnus, who passed ENGR 233 with A+. 2 To tackle the problem of finding the volume of concrete work, consider the dome as a lamina with the thickness of 3 20 cm and neglect the interaction between the column and the dome, therefore the total volume of concrete work would be the sum of concrete work for the column and the dome. Note that the critical value for R*can be found graphically by plotting the f = 2,000,000 - Price Total against different R*. Critical values of R* is where f = 0.