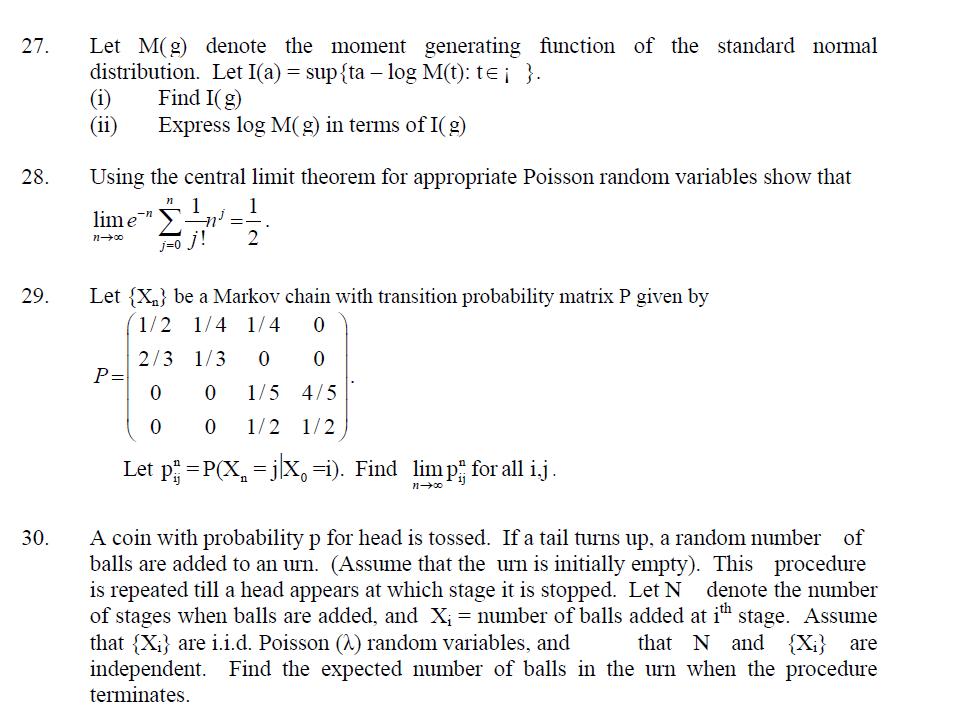
26. Let X and Y be two independent random variables such that X is uniformly distributed on [0, 1] and Y has a discrete uniform distribution on {0, 1, 2,L, n-1}, that is, 1 P(Y=k)=n if k=0,1,L,n-1, 0, otherwise. Define Z = X + Y. Show that Z is uniformly distributed on [0, n]. 27. 28. 29. Let M(g) denote the moment generating function of the standard normal distribution. Let I(a) = sup {ta-log M(t): te }. (i) (ii) Find I(g) Express log M(g) in terms of I(g) Using the central limit theorem for appropriate Poisson random variables show that lime " 11-00 j=0 1 2 Let {X} be a Markov chain with transition probability matrix P given by 1/2 1/4 1/4 0 2/3 1/3 00 P= 0 0 1/5 4/5 30. 0 0 1/2 1/2 == Let p = P(X =jX =i). Find limp for all i.j. 11-00 A coin with probability p for head is tossed. If a tail turns up, a random number of balls are added to an urn. (Assume that the urn is initially empty). This procedure is repeated till a head appears at which stage it is stopped. Let N denote the number of stages when balls are added, and X = number of balls added at ith stage. Assume that {X} are i.i.d. Poisson (X) random variables, and that N and {X} are independent. Find the expected number of balls in the urn when the procedure terminates.