$3\mathrm{.}10".$ When an insoluble bubble rises in a deep pool of liquid, its volume increases according to the ideal gas law. However, when a soluble bubble rises from deep submersion, there is a competing action of dissolution that tends to reduce size. Under practical conditions, it has been proved $($Rice $1982)$ that the mass transfer coefficient $(k_c)$ for spherical particles $($or bubbles$)$ in free fall $($or free$-$rise$)$ is substantially constant. Thus, for sparingly soluble bubbles released from rest, the following material balance is applicable:

$\frac{d(C*(\frac{4}{3})R^3)}{dt}=-k_c*C^{**}*4R^2(t)$

where $C=\frac{P}{R_g}T$ is the $($ideal$)$ molar density of gas, $C^{**}$ is molar solubility of gas in liquid, and $R(t)$ is the changing bubble radius. The pressure at a distance $z$ from the top liquid surface is $P=P_A+{}_{L}gz,$ and the rise velocity is assumed to be quasi$-$steady and follows the intermediate law according to Rice and Littlefield $(1987)$ to give a linear relation between speed and size

$\frac{dz}{dt}=U=(\frac{2g}{15v^{\frac{1}{2}}}{)}^{\frac{2}{3}}*2R(t)=*R(t)$:

note $<mn\; id="EditorElement\_3974497">0</mn>$ for rising bubble.

where $g$ is gravitational acceleration, and $v$ is liquid kinematic viscosity.

a$.$ Show that a change of variables allows the material balance to be written as

null

$R\frac{dR}{dP}+\frac{1}{3}\frac{R^2}{P}=-\frac{}{P}$

where

$=\frac{k_c{R}_{g}T{C}^{**}}{{}_{L}g}<mn\; id="EditorElement\_3974064">0</mn>$ since $<mn\; id="EditorElement\_3974082">0</mn>$

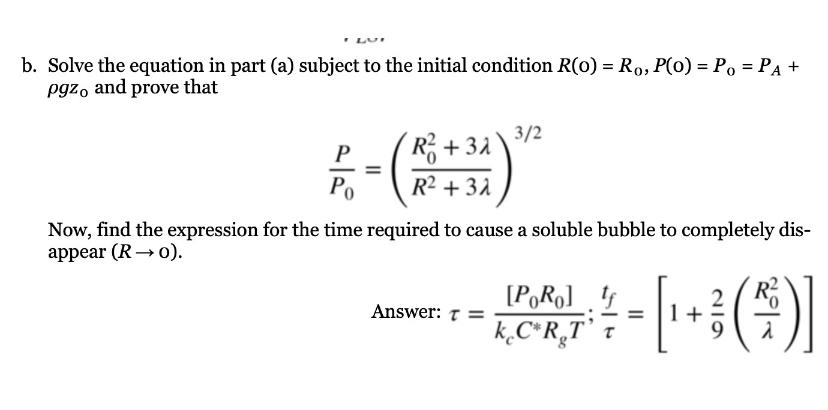
b$.$ Solve the equation in part $($a$)$ subject to the initial condition $R(o)=R_0,P(o)=P_o=P_A+g{z}_0$ and prove that

$\frac{P}{P_0}=(\frac{R_0^2+3}{R^2+3}{)}^{\frac{3}{2}}$

Now, find the expression for the time required to cause a soluble bubble to completely disappear $(R0).$

Answer: $=\frac{[P_0{R}_0]}{k_c{C}^{**}{R}_{g}T}$;$\frac{t_f}{}=[1+\frac{2}{9}(\frac{R_0^2}{})]$

Only want b time expressions $!$ Please show how to get them $!$