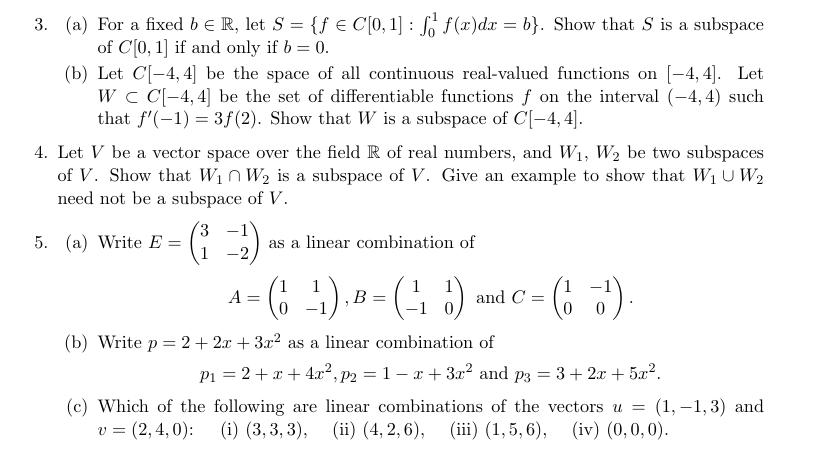
: 3. (a) For a fixed b = R, let S = { = C[0,1] f f(x)dx = b}. Show that S is a subspace of C[0, 1] if and only if b = 0. (b) Let C-4,4] be the space of all continuous real-valued functions on [-4,4]. Let WC C-4,4] be the set of differentiable functions f on the interval (-4, 4) such that f'(-1)=3f(2). Show that W is a subspace of C[-4,4]. 4. Let V be a vector space over the field R of real numbers, and W1, W be two subspaces of V. Show that Wn W is a subspace of V. Give an example to show that WU W need not be a subspace of V. as a linear combination of 3 5. (a) Write E= -2 A = (61), B = ( 4 ) and C = = (6J). 1 (b) Write p 2+2x+3x2 as a linear combination of = P =2+x+4x2, p2 = 1-x+3x and p3 = 3+2x+5x2. (1,-1,3) and (c) Which of the following are linear combinations of the vectors u = v = (2,4,0): (i) (3,3,3), (ii) (4, 2, 6), (iii) (1,5,6), (iv) (0,0,0).