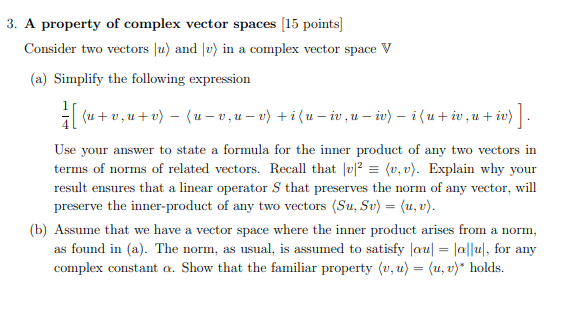
3. A property of complex vector spaces [15 points] Consider two vectors |u) and (v) in a complex vector space V (a) Simplify the following expression [+ [(u+v,u+v) (uvu-v) +i (uiv, u iv) i (u+ivu+iv)]. Use your answer to state a formula for the inner product of any two vectors in terms of norms of related vectors. Recall that |v|2 = (v, v). Explain why your result ensures that a linear operator S that preserves the norm of any vector, will preserve the inner-product of any two vectors (Su, Sv) = (u, v). (b) Assume that we have a vector space where the inner product arises from a norm, as found in (a). The norm, as usual, is assumed to satisfy |au| = |a||u, for any complex constant a. Show that the familiar property (v, u) = (u, v)* holds.