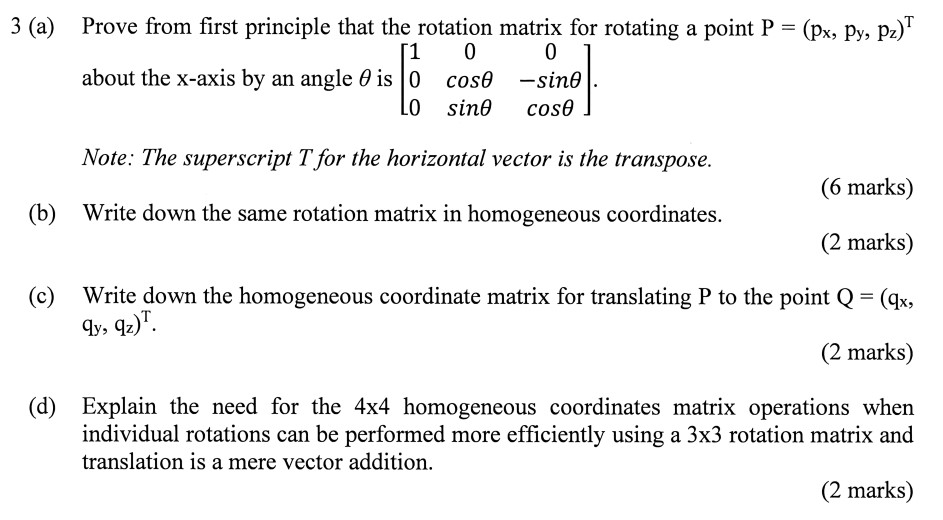
3 (a) Prove from first principle that the rotation matrix for rotating a point P = (px, py, pz)T [1 0 0 about the x-axis by an angle is 0 cose -sine LO sine cose Note: The superscript T for the horizontal vector is the transpose. (6 marks) (b) Write down the same rotation matrix in homogeneous coordinates. (2 marks) (c) Write down the homogeneous coordinate matrix for translating P to the point Q = (qx, qy, qz)T. (2 marks) (d) Explain the need for the 4x4 homogeneous coordinates matrix operations when individual rotations can be performed more efficiently using a 3x3 rotation matrix and translation is a mere vector addition. (2 marks)