3. Assume the Black-Scholes model with no interest, ie. t = 1 for all t 0, and H=2, = 1, So = 1. (a) Let X be the contact that pays S3, at time T. Denote the price of X at time t by C = C(St, t) for a function C(x, t). Write the Black-Scholes PDE for the price, specifying the boundary condition. (b) Calculate C, by taking expectation under the EMM. Hence provide the solution to the PDE in (a). (c) Give the self-financing replicating portfolio (a, b), and verify that it is self-financing and replicating. (d) Suppose you want to price a contract that pays Y = (X-1)+ = (S-1)+ at time T. Write the PDEs for the price D, of Y at time t, and give the boundary condition. Explain the difference with the PDE in (a). (e) Let a self-financing portfolio have a(t) shares at time t, for some given differentiable function a. Find b(t) the money in savings account by using the self-financing property of the portfolio. Hence give the contract this portfolio replicates at time T.

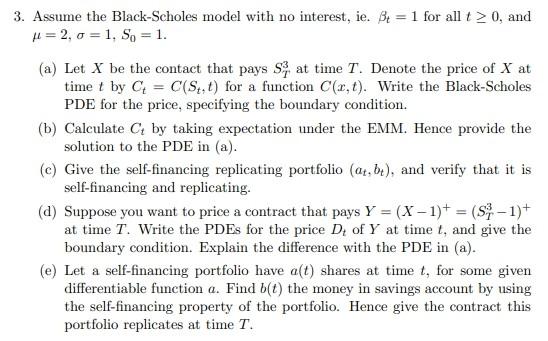
3. Assume the Black-Scholes model with no interest, ie. t=1 for all t0, and =2,=1,S0=1. (a) Let X be the contact that pays ST3 at time T. Denote the price of X at time t by Ct=C(St,t) for a function C(x,t). Write the Black-Scholes PDE for the price, specifying the boundary condition. (b) Calculate Ct by taking expectation under the EMM. Hence provide the solution to the PDE in (a). (c) Give the self-financing replicating portfolio (at,bt), and verify that it is self-financing and replicating. (d) Suppose you want to price a contract that pays Y=(X1)+=(ST31)+ at time T. Write the PDEs for the price Dt of Y at time t, and give the boundary condition. Explain the difference with the PDE in (a). (e) Let a self-financing portfolio have a(t) shares at time t, for some given differentiable function a. Find b(t) the money in savings account by using the self-financing property of the portfolio. Hence give the contract this portfolio replicates at time T