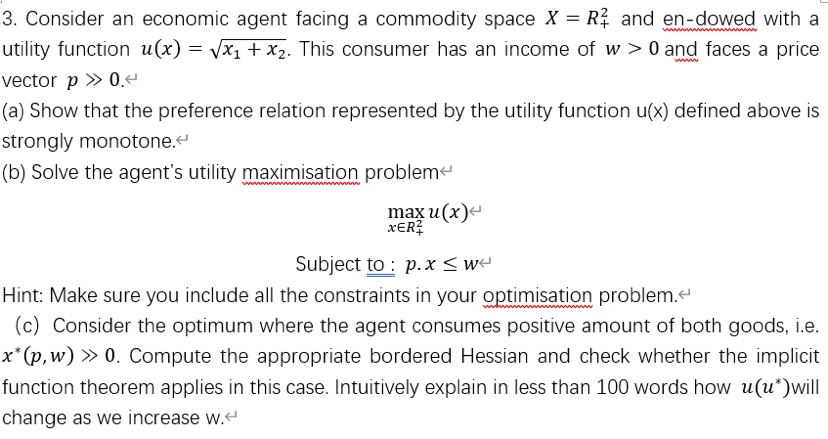3. Consider an economic agent facing a commodity space X=R+2 and en-dowed with a utility function u(x)=x1+x2. This consumer has an income of w>0 and faces a price vector p0. (a) Show that the preference relation represented by the utility function u(x) defined above is strongly monotone. (b) Solve the agent's utility maximisation problem maxxR+2u(x) Subject to : p.xw Hint: Make sure you include all the constraints in your optimisation problem. (c) Consider the optimum where the agent consumes positive amount of both goods, i.e. x(p,w)0. Compute the appropriate bordered Hessian and check whether the implicit function theorem applies in this case. Intuitively explain in less than 100 words how u(u) will change as we increase w. 3. Consider an economic agent facing a commodity space X=R+2 and en-dowed with a utility function u(x)=x1+x2. This consumer has an income of w>0 and faces a price vector p0. (a) Show that the preference relation represented by the utility function u(x) defined above is strongly monotone. (b) Solve the agent's utility maximisation problem maxxR+2u(x) Subject to : p.xw Hint: Make sure you include all the constraints in your optimisation problem. (c) Consider the optimum where the agent consumes positive amount of both goods, i.e. x(p,w)0. Compute the appropriate bordered Hessian and check whether the implicit function theorem applies in this case. Intuitively explain in less than 100 words how u(u) will change as we increase w