Question: 3. Consider the two lines L: r = <1,1,0>+t <1,-1,2> and L2: r = < 2,0,2>+s . (a) Show that the line L1 and

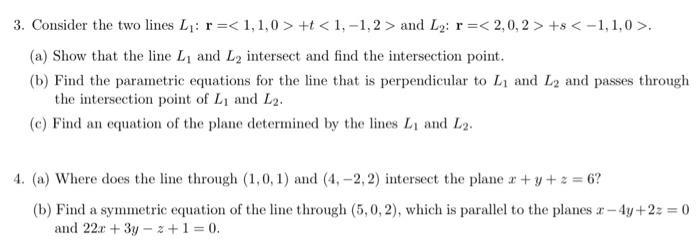
3. Consider the two lines L: r = +t and L2: r = < 2,0,2>+s . (a) Show that the line L1 and L2 intersect and find the intersection point. (b) Find the parametric equations for the line that is perpendicular to L1 and L2 and passes through the intersection point of L1 and L2. (c) Find an equation of the plane determined by the lines L1 and L2. 4. (a) Where does the line through (1,0, 1) and (4, -2, 2) intersect the plane x+y+2=6? (b) Find a symmetric equation of the line through (5,0,2), which is parallel to the planes x-4y+22=0 and 22x+3y-2+1=0.

Step by Step Solution

There are 3 Steps involved in it

1 Expert Approved Answer

Step: 1 Unlock

a To show that the lines L and L intersect we need to find the values of t and s for which the equations of the lines are satisfied simultaneously The ... View full answer

Question Has Been Solved by an Expert!

Get step-by-step solutions from verified subject matter experts

Step: 2 Unlock

Step: 3 Unlock

Students Have Also Explored These Related Mathematics Questions!

Acme Inc. just paid an annual dividend of 1.67. You expect Acme to make a big push into the Internet-of-Things (IoT) space during the next four (4) years. As a result, you expect Acme's dividend...

QUIZ... Let D be a poset and let f : D D be a monotone function. (i) Give the definition of the least pre-fixed point, fix (f), of f. Show that fix (f) is a fixed point of f. [5 marks] (ii) Show that...

Data gistrun sending inside a pack fills in as conventional allowing subordinate rules to execute on progressive clock cycles. Correspondence between gatherings conventionally causes a surprising...

I am very confused by this question and where to even start? Are you able to do it and show the work so that I can see how it is done?

I need solution Two lines in R' . L1 and L2, are given by the following sets of symmetric equations: I 1 y z + '2 L : z ' : ' 2 3 5 L : = Ii = i. 2 I y 3 (a) [8 points] Determine if L: and L2 are...

20. Passes through P = (3,5, -9) and is parallel to the xz-plane 39 In Exercises 21-24, find an equation of the plane passing through the three points given. 21. P = (2, -1,4), Q = (1, 1, 1), R= (3,...

1. Given the vectors u = i+j+2k = (1,1,2), v 2 2i j+k = {2. l, 1}, and w = i+3k = (1,0, 3) nd: (a (3 points) The dot product 11 - v ( (3 points) The cross product v x w (c) (3 points) The projection...

Let Z = R+. Consider the following lotteries: L I 1 = [$100] L I 2 = 0.75 [$150] 0.25 [$1] L II 1 = 1/3 [$100] 2/3 [$1] L II 2 = 0.25 [$150] 0.75 [$1] (a) Depict each of the lotteries L I 1 , L I 2 ,...

NET Go to www.surveypro.com. Click on pricing. Write a brief report that describes how prices are charged to someone wishing to use this service to host a survey. What happens as the desired sample...

Latrobe Corporation holds as a long-term investment available-for-sale stock securities costing $72,000. At December 31, 2012, the fair value of the securities is $66,000. Prepare the adjusting entry...

A Canadian subsidiary of a U . S . parent frm is instructed to bill an export to the parent in U . S . dollars. The Canadian subsidiary records the accounts recelvable in Canadian dollars and notes a...

Replacing incandescent lights with energy-efficient fluorescent lights can reduce the lighting energy consumption to one-fourth of what it was before. The energy consumed by the lamps is eventually...

An insulated pistoncylinder device initially contains 0.02 m3 of saturated liquidvapor mixture of water with a quality of 0.1 at 100°C. Now some ice at -18°C is dropped into the cylinder. If...

Consider a homeowner who is replacing his 25-yearold natural gas furnace that has an efficiency of 55 percent. The homeowner is considering a conventional furnace that has an efficiency of 82 percent...

The early steam engines were driven by the atmospheric pressure acting on the piston fitted into a cylinder filled with saturated steam. A vacuum was created in the cylinder by cooling the cylinder...

J. Chadwick discovered the neutron by bombarding 9.4Be with the popular projectile of the day, alpha particles. (a) If one of the reaction products was the then unknown neutron, what was the other...

6 Theory of Machines 5. A shaft carries five masses A, B, C, D and E which revolve at the same radius in planes which are equidistant from one another. The magnitude of the masses in planes A, C and...

Evaluate the limit by first recognizing the sum as a Riemann sum for a function defined on [0, 1]. i-1 n

The graph of is shown. Evaluate each integral by interpreting it in terms of areas. a. b. c. d. f(x) dx f(x) dx f(x) dx f(x) dx 0 4 6

If Where is a continuous function, find f(4). f(t) dt. xsin TX =

Use your knowledge of subscripts and addresses to complete the following matrix, given a12 = 9, a21 = 4, a13 = 5, a31 = 2, a23 = 7, and a32 = 3. Since the subscripts are always given in row-column...

A company with four retail stores has 35 TVs t, 60 stereos s, 55 VCRs (videocassette recorders) v, and 45 camcorders c in store 1; 80t, 65s, 50v, and 38c in store 2; 29t, 36s, 24v, and 32c in store...

Find the break-even point for each of the following monopolistic firms, given (a) R(x) = x + 22x C(x)=7x+36 (c) R(x) = -2x + 85x == C(x) 11x+420 = (b) R(x)=-5x + 163x C(x)=23x + 800 (d) R(x)=-3x+201x...