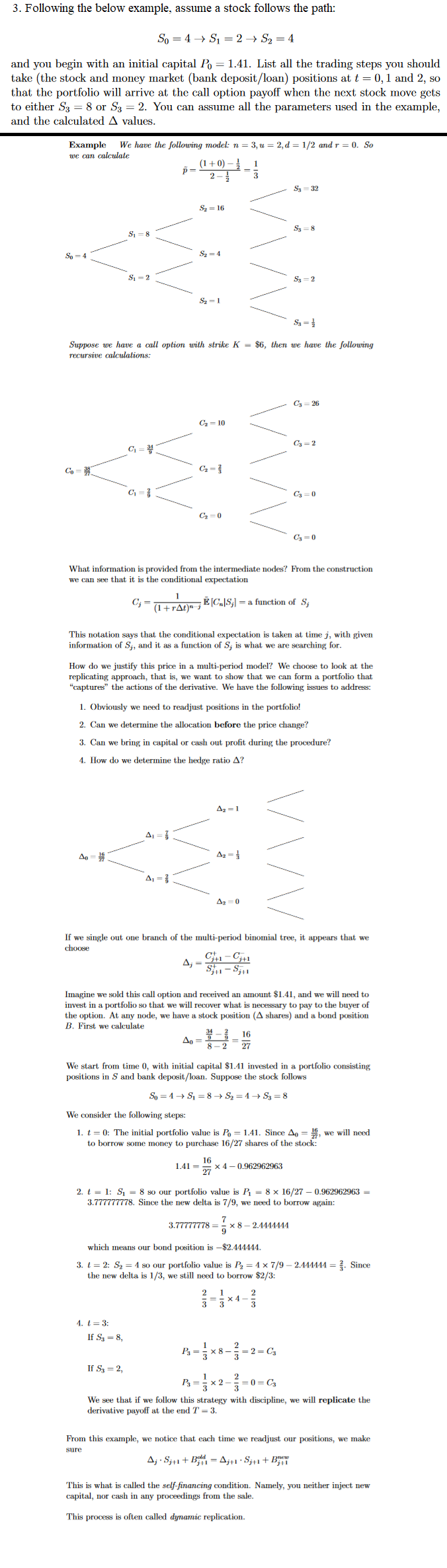
3. Following the below example, assume a stock follows the path: So = 4 Si = 2 S2 = 4 and you begin with an initial capital Po = 1.41. List all the trading steps you should take the stock and money market (bank deposit/loan) positions at t = 0,1 and 2, so that the portfolio will arrive at the call option payoff when the next stock move gets to either S3 = 8 or S3 = 2. You can assume all the parameters used in the example, and the calculated A values. Example We have the following model: n = 3, u = 2, d = 1/2 and r = 0. So we can calculate (1+0) 1 p= 2 3 Sg = 32 S = 16 Ss=8 S-8 So = 4 S = 4 S = 2 S3 = 2 Sz=1 S Suppose we have a call option with strike K = $6, then we have the following recursive calculations: C = 26 C = 10 C=2 C = Co- C-3 C=0 C2-0 C3= 0 What information is provided from the intermediate nodes? From the construction we can see that it is the conditional expectation C;= (1+rAt)" ; ECS; - a function of S; This notation says that the conditional expectation is taken at time j, with given information of Sj, and it as a function of S, is what we are searching for. How do we justify this price in a multi-period model? We choose to look at the replicating approach, that is, we want to show that we can form a portfolio that "captures" the actions of the derivative. We have the following issues to address: 1. Obviously we need to readjust positions in the portfolio! 2. Can we determine the allocation before the price change? 3. Can we bring in capital or cash out profit during the procedure? 4. How do we determine the hedge ratio A? Ay = 1 A1 = 1 - Ay - 1 A = 3 A2 - 0 If we single out one branch of the multi-period binomial tree, it appears that we choose C+-C+1 , - 5:1-5; Imagine we sold this call option and received an amount $1.41, and we will need to invest in a portfolio so that we will recover what is necessary to pay to the buyer of the option. At any node, we have a stock position (A shares) and a bond position B. First we calculate A = We start from time 0, with initial capital $1.41 invested in a portfolio consisting positions in Sand bank deposit/loan. Suppose the stock follows So = 1 S = 8 S = 1 S3 = 8 We consider the following steps: 1. t= 0: The initial portfolio value is Po=1.41. Since Ao = we will need to borrow some money to purchase 16/27 shares of the stock: 16 1.41 - x 40.962962963 2. t = 1: S = 8 so our portfolio value is Pi = 8 x 16/27 - 0.962962963 = 3.777777778. Since the new delta is 7/9, we need to borrow again: 3.77777778 = x 8-2.4444444 which means our bond position is $2.444444. 3. 1 = 2: S, = 1 so our portfolio value is P = 4 x 7/9 -2.441411 = . Since the new delta is 1/3, we still need to borrow $2/3: 2 2 x 4 3 1. L = 3: If S3 = 8, P3 Ps= 3x8 = 2 = Cg 2 If S3 = 2, P = 2 3 We see that if we follow this strategy with discipline, we will replicate the derivative payoff at the end T = 3. From this example, we notice that each time we readjust our positions, we make sure 4;. Sj+1+B=A+1S;+1+BET This is what is called the self-financing condition. Namely, you neither inject new capital, nor cash in any proceedings from the sale. This process is often called dynamic replication. 3. Following the below example, assume a stock follows the path: So = 4 Si = 2 S2 = 4 and you begin with an initial capital Po = 1.41. List all the trading steps you should take the stock and money market (bank deposit/loan) positions at t = 0,1 and 2, so that the portfolio will arrive at the call option payoff when the next stock move gets to either S3 = 8 or S3 = 2. You can assume all the parameters used in the example, and the calculated A values. Example We have the following model: n = 3, u = 2, d = 1/2 and r = 0. So we can calculate (1+0) 1 p= 2 3 Sg = 32 S = 16 Ss=8 S-8 So = 4 S = 4 S = 2 S3 = 2 Sz=1 S Suppose we have a call option with strike K = $6, then we have the following recursive calculations: C = 26 C = 10 C=2 C = Co- C-3 C=0 C2-0 C3= 0 What information is provided from the intermediate nodes? From the construction we can see that it is the conditional expectation C;= (1+rAt)" ; ECS; - a function of S; This notation says that the conditional expectation is taken at time j, with given information of Sj, and it as a function of S, is what we are searching for. How do we justify this price in a multi-period model? We choose to look at the replicating approach, that is, we want to show that we can form a portfolio that "captures" the actions of the derivative. We have the following issues to address: 1. Obviously we need to readjust positions in the portfolio! 2. Can we determine the allocation before the price change? 3. Can we bring in capital or cash out profit during the procedure? 4. How do we determine the hedge ratio A? Ay = 1 A1 = 1 - Ay - 1 A = 3 A2 - 0 If we single out one branch of the multi-period binomial tree, it appears that we choose C+-C+1 , - 5:1-5; Imagine we sold this call option and received an amount $1.41, and we will need to invest in a portfolio so that we will recover what is necessary to pay to the buyer of the option. At any node, we have a stock position (A shares) and a bond position B. First we calculate A = We start from time 0, with initial capital $1.41 invested in a portfolio consisting positions in Sand bank deposit/loan. Suppose the stock follows So = 1 S = 8 S = 1 S3 = 8 We consider the following steps: 1. t= 0: The initial portfolio value is Po=1.41. Since Ao = we will need to borrow some money to purchase 16/27 shares of the stock: 16 1.41 - x 40.962962963 2. t = 1: S = 8 so our portfolio value is Pi = 8 x 16/27 - 0.962962963 = 3.777777778. Since the new delta is 7/9, we need to borrow again: 3.77777778 = x 8-2.4444444 which means our bond position is $2.444444. 3. 1 = 2: S, = 1 so our portfolio value is P = 4 x 7/9 -2.441411 = . Since the new delta is 1/3, we still need to borrow $2/3: 2 2 x 4 3 1. L = 3: If S3 = 8, P3 Ps= 3x8 = 2 = Cg 2 If S3 = 2, P = 2 3 We see that if we follow this strategy with discipline, we will replicate the derivative payoff at the end T = 3. From this example, we notice that each time we readjust our positions, we make sure 4;. Sj+1+B=A+1S;+1+BET This is what is called the self-financing condition. Namely, you neither inject new capital, nor cash in any proceedings from the sale. This process is often called dynamic replication