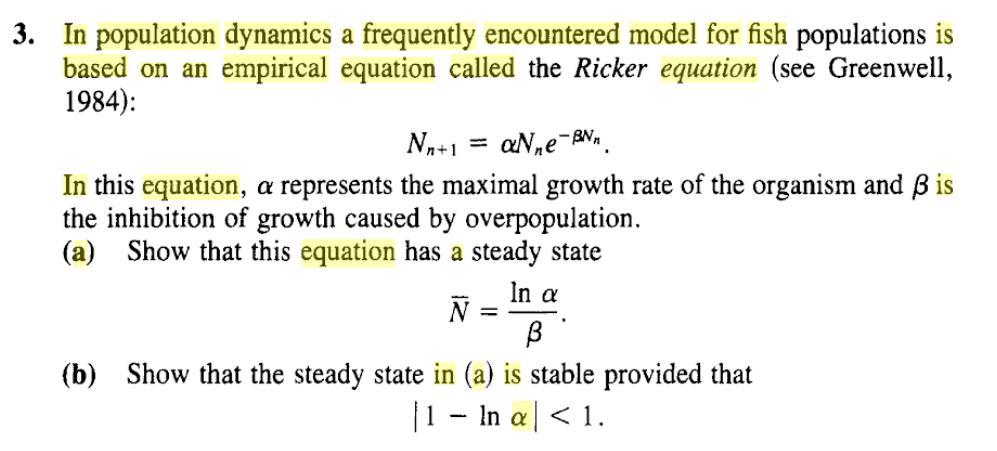
3. In population dynamics a frequently encountered model for fish populations is based on an empirical equation called the Ricker equation (see Greenwell, 1984): Nn+1 =aNne-BNn In this equation, a represents the maximal growth rate of the organism and 3 is the inhibition of growth caused by overpopulation. (a) Show that this equation has a steady state In a N B (b) Show that the steady state in (a) is stable provided that |1 In a | < 1.