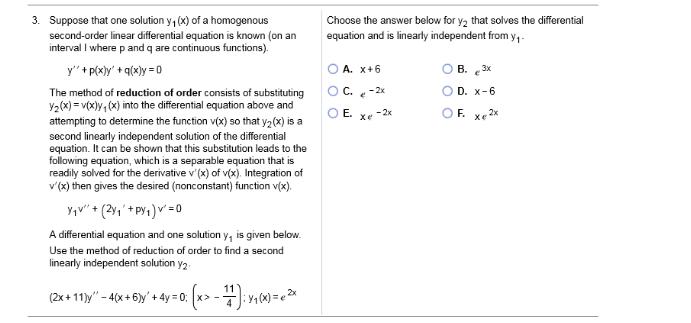
3. Suppose that one solution y, (x) of a homogenous second-order linear differential equation is known (on an interval I where p and q are continuous functions). Choose the answer below for y, that solves the differential equation and is linearly independent from y,- O A. X+6 OC. -2x y" + p(x}y' + q(x]y = 0 B. 3x O D. x-6 The method of reduction of order consists of substituting Y2(x) = v(x)y, (x) into the differential equation above and attempting to determine the function v(x) so that y2 (x) is a second linearly independent solution of the differential equation. It can be shown that this substitution leads to the following equation, which is a separable equation that is readily solved for the derivative v'(x) of v(x). Integration of v'(x) then gives the desired (nonconstant) function v(x). O E. -2x xe F. xe 2x Y,v' + (2y, + Py1)v =0 A differential equation and one solution y, is given below. Use the method of reduction of order to find a second linearly independent solution y2 (2x + 11)y" - 4(x+6)y'+ 4y 0;