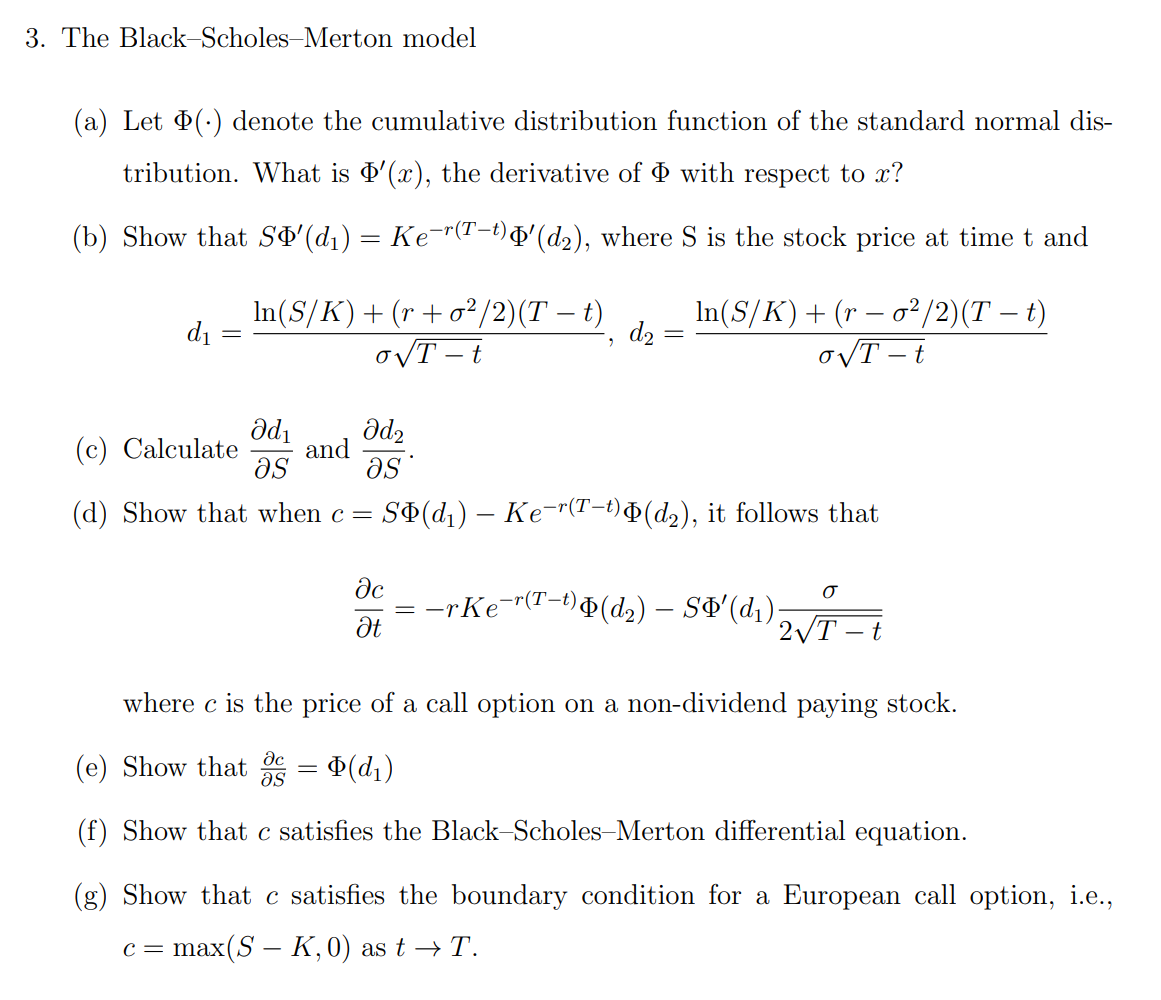
3. The Black-Scholes-Merton model (a) Let () denote the cumulative distribution function of the standard normal dis- tribution. What is '(x), the derivative of with respect to x? (b) Show that So'(d1) = Ke"(Tt) '(d2), where S is the stock price at time t and In(S/K)+(r+o/2)(T t) - In(S/K)+(ro/2)(T t) d = d2 = " t oT-t (c) Calculate and as Jd2 as (d) Show that when c = - = S(d) Ke(Tt)(d2), it follows that t rKe(Tt) (d2) S'(d); where c is the price of a call option on a non-dividend paying stock. 2T t (e) Show that as = = (d1) (f) Show that c satisfies the Black-Scholes-Merton differential equation. (g) Show that c satisfies the boundary condition for a European call option, i.e., c = max(SK, 0) as t T.