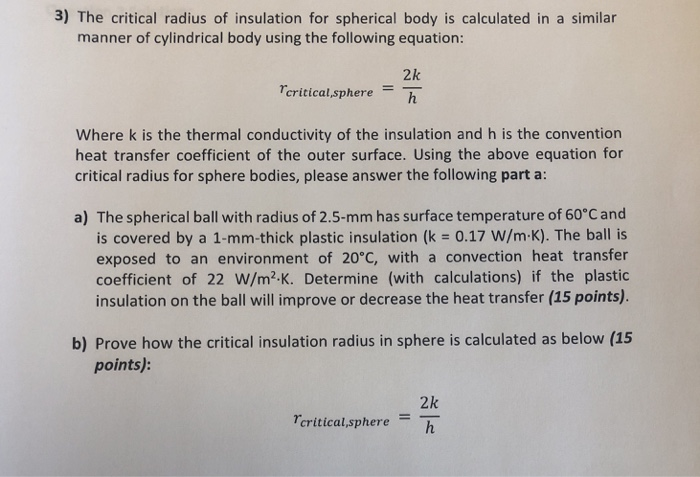
3) The critical radius of insulation for spherical body is calculated in a similar manner of cylindrical body using the following equation: Tcritical sphere = 2k h Where k is the thermal conductivity of the insulation and h is the convention heat transfer coefficient of the outer surface. Using the above equation for critical radius for sphere bodies, please answer the following part a: a) The spherical ball with radius of 2.5-mm has surface temperature of 60C and is covered by a 1-mm-thick plastic insulation (k = 0.17 W/m-K). The ball is exposed to an environment of 20C, with a convection heat transfer coefficient of 22 W/m.K. Determine (with calculations) if the plastic insulation on the ball will improve or decrease the heat transfer (15 points). b) Prove how the critical insulation radius in sphere is calculated as below (15 points): Tcritical sphere = 2k h