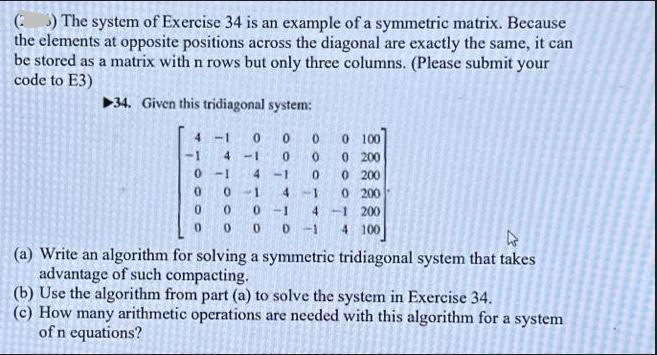
(3) The system of Exercise 34 is an example of a symmetric matrix. Because the elements at opposite positions across the diagonal are exactly the same, it can be stored as a matrix with n rows but only three columns. (Please submit your code to E3) 34. Given this tridiagonal system: 4 0 0 100 1 0 0 200 0 -1 4 -1 0 0 200 0 0 1 4-1 0 200 0 0 0-1 4-1 200 0 0 0 0-1 4 100 -1 0 4-1 0 0 (a) Write an algorithm for solving a symmetric tridiagonal system that takes advantage of such compacting. (b) Use the algorithm from part (a) to solve the system in Exercise 34. (c) How many arithmetic operations are needed with this algorithm for a system of n equations?