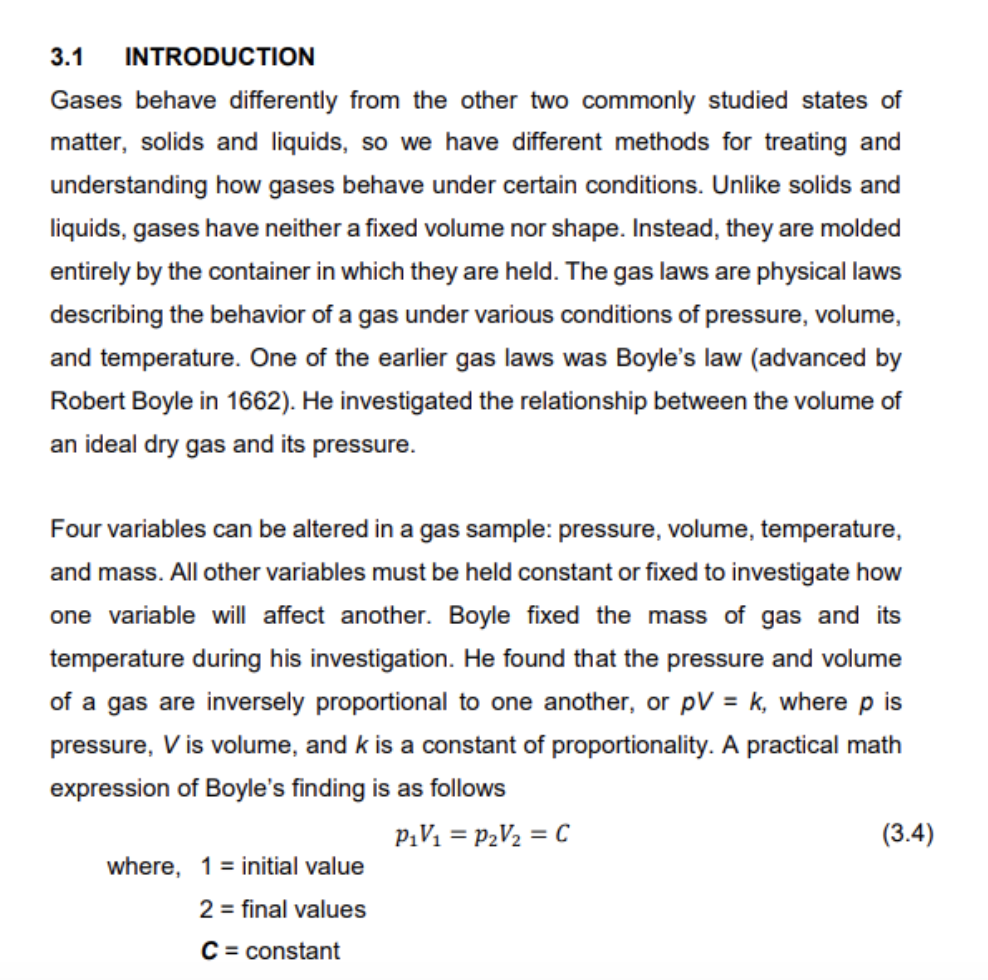
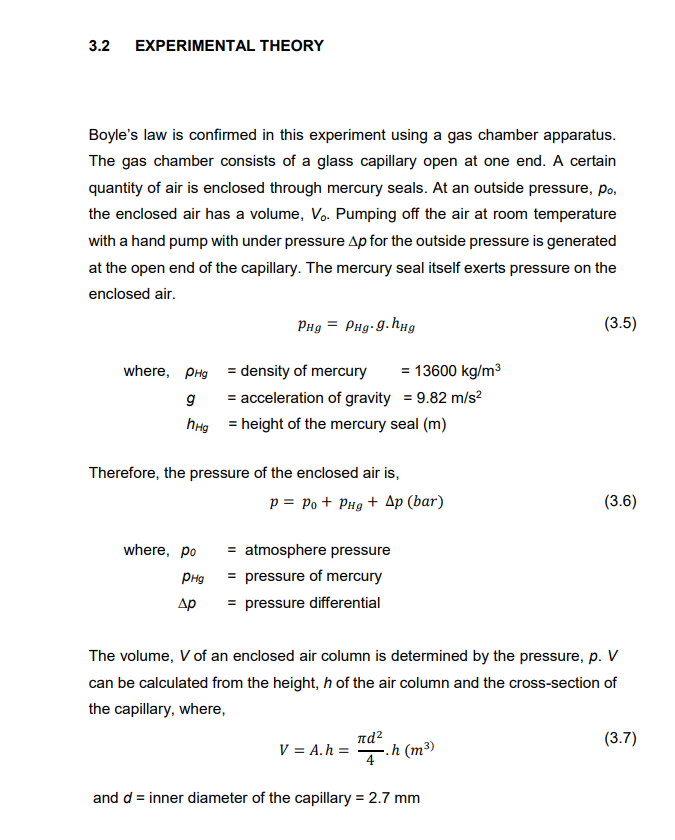
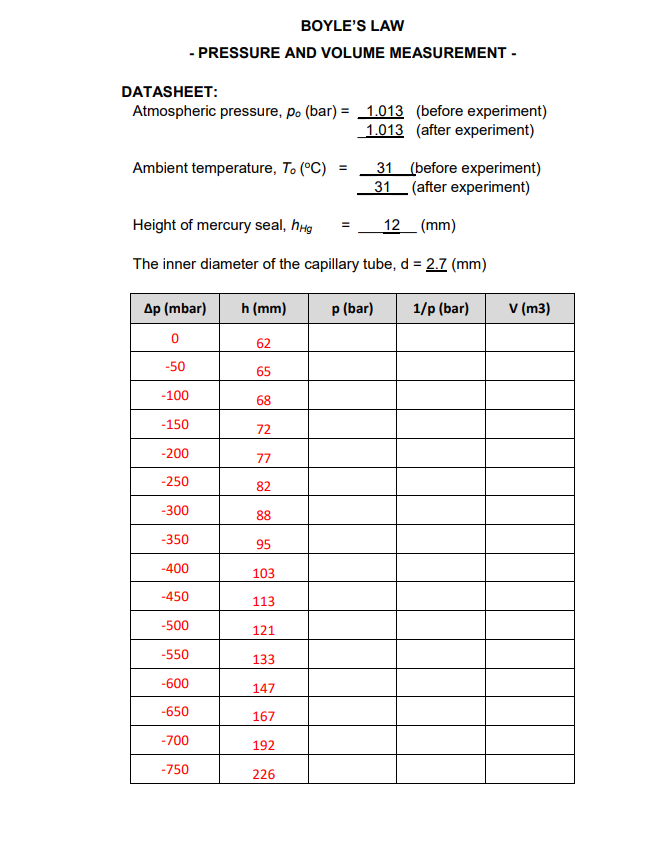
3.1 INTRODUCTION Gases behave differently from the other two commonly studied states of matter, solids and liquids, so we have different methods for treating and understanding how gases behave under certain conditions. Unlike solids and liquids, gases have neither a fixed volume nor shape. Instead, they are molded entirely by the container in which they are held. The gas laws are physical laws describing the behavior of a gas under various conditions of pressure, volume, and temperature. One of the earlier gas laws was Boyle's law (advanced by Robert Boyle in 1662). He investigated the relationship between the volume of an ideal dry gas and its pressure. Four variables can be altered in a gas sample: pressure, volume, temperature, and mass. All other variables must be held constant or fixed to investigate how one variable will affect another. Boyle fixed the mass of gas and its temperature during his investigation. He found that the pressure and volume of a gas are inversely proportional to one another, or pV=k, where p is pressure, V is volume, and k is a constant of proportionality. A practical math expression of Boyle's finding is as follows where,12CC=initialvaluep1V1=p2V2=C=finalvaluesconstant 3.2 EXPERIMENTAL THEORY Boyle's law is confirmed in this experiment using a gas chamber apparatus. The gas chamber consists of a glass capillary open at one end. A certain quantity of air is enclosed through mercury seals. At an outside pressure, p0, the enclosed air has a volume, V0. Pumping off the air at room temperature with a hand pump with under pressure p for the outside pressure is generated at the open end of the capillary. The mercury seal itself exerts pressure on the enclosed air. where,HgghHgpHg=HgghHg=densityofmercury=13600kg/m3=accelerationofgravity=9.82m/s2=heightofthemercuryseal(m) Therefore, the pressure of the enclosed air is, p=p0+pHg+p(bar) where, p0= atmosphere pressure pHg= pressure of mercury p= pressure differential The volume, V of an enclosed air column is determined by the pressure, p. V can be calculated from the height, h of the air column and the cross-section of the capillary, where, V=Ah=4d2h(m3) and d= inner diameter of the capillary =2.7mm DATASHEET: Atmospheric pressure, p (bar) =1.0131.013 (before experiment) Ambient temperature, T0(C)=3131 (before experiment) Height of mercury seal, hHg=12(mm) The inner diameter of the capillary tube, d=2.7(mm)