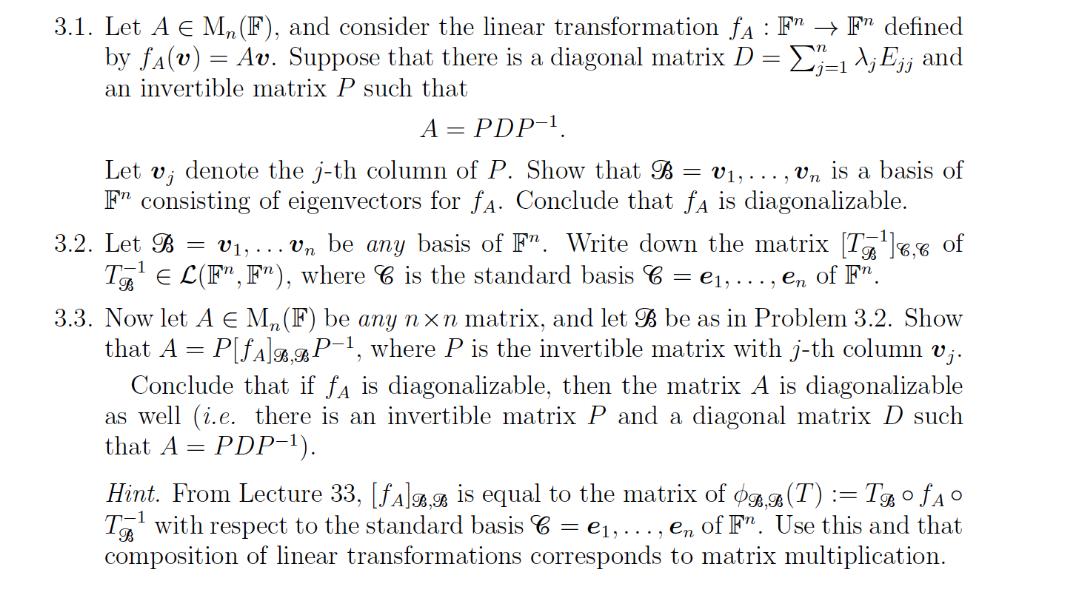
3.1. Let A Mn (F), and consider the linear transformation fA F F defined by f(v) = Av. Suppose that there is a diagonal matrix D = 1 AjEjj and an invertible matrix P such that A = PDP- Let vj denote the j-th column of P. Show that B = V1,..., Un is a basis of F consisting of eigenvectors for fA. Conclude that fa is diagonalizable. B 3.2. Let B = v,... Un be any basis of F. Write down the matrix [T], of T L(F,F), where is the standard basis = e,..., en of Fr. 3.3. Now let AE M (F) be any nxn matrix, and let B be as in Problem 3.2. Show that A = P[fa],P-1, where P is the invertible matrix with j-th column vj. Conclude that if f is diagonalizable, then the matrix A is diagonalizable as well (i.e. there is an invertible matrix P and a diagonal matrix D such that A = = PDP-). Hint. From Lecture 33, [fa], is equal to the matrix of (T) = T Twith respect to the standard basis C = ,..., en of F. Use this and that composition of linear transformations corresponds to matrix multiplication. O