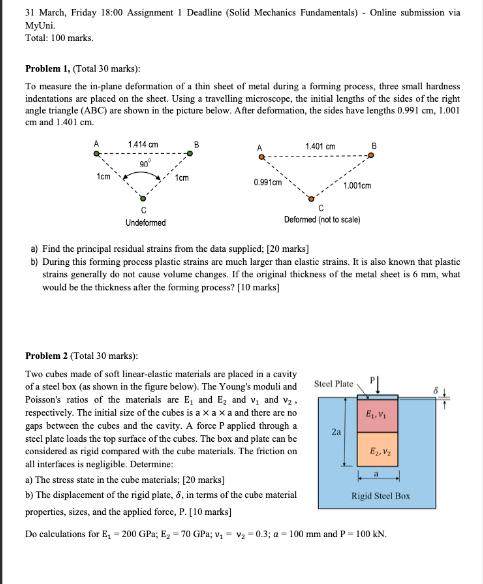
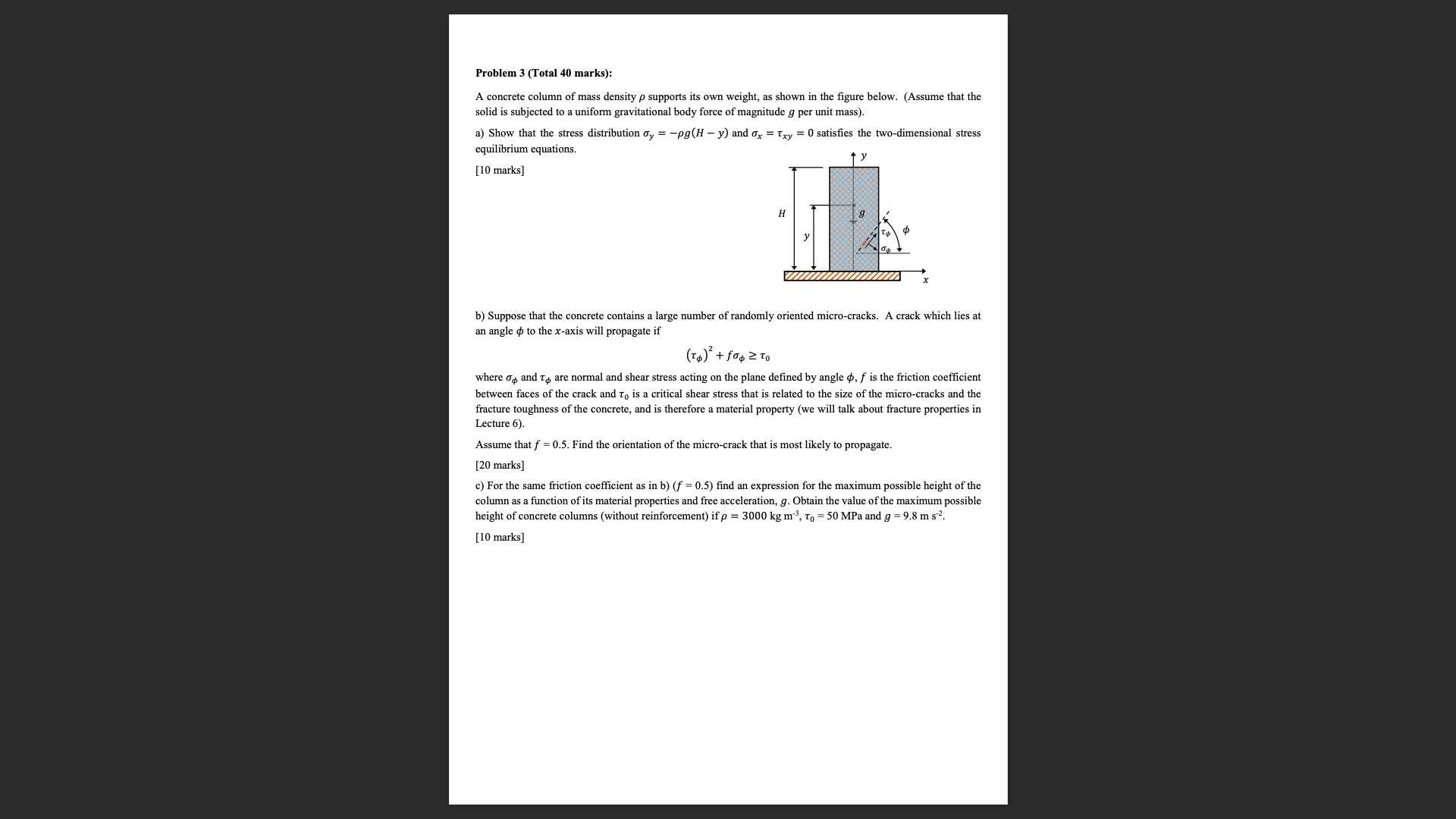
31 March, Friday 18:00 Assignment | Deadline (Solid Mechanics Fundamentals) Online submission via MyUni. Total: 100 marks. Problem 1, (Total 30 marks): To measure the in-plane deformation of a thin sheet of metal during a forming process, three small hardness indentations are placed on the sheet. Using a travelling microscope, the initial lengths of the sides of the right angle triangle (ABC) are shown in the picture below. After deformation, the sides have lengths 0.991 cm, 1.001 cm and 1.401 cm. 1414 am 90 1.401 cm B 1cm 1cm 0.991am 1.001cm Undeformed Deformed (not to scale) a) Find the principal residual strains from the data supplied; [20 marks] b) During this forming process plastic strains are much larger than elastic strains. It is also known that plastic strains generally do not cause volume changes. If the original thickness of the metal sheet is 6 mm, what would be the thickness after the forming process? [10 marks] Problem 2 (Total 30 marks): Two cubes made of soft linear-elastic materials are placed in a cavity of a steel box (as shown in the figure below). The Young's moduli and Poisson's ratios of the materials are E, and E and v and v. respectively. The initial size of the cubes is a xa xa and there are no gaps between the cubes and the cavity. A force P applied through a steel plate loads the top surface of the cubes. The box and plate can be considered as rigid compared with the cube materials. The friction on all interfaces is negligible. Determine: a) The stress state in the cube materials; [20 marks] b) The displacement of the rigid plate, 5, in terms of the cube material properties, sizes, and the applied force, P. [10 marks] Steel Plate E.V Za E2. Vz Rigid Steel Box Do calculations for E =200 GPa; E-70 GPa; v =v2 -0.3; a 100 mm and P = 100 kN. Problem 3 (Total 40 marks): A concrete column of mass density p supports its own weight, as shown in the figure below. (Assume that the solid is subjected to a uniform gravitational body force of magnitude g per unit mass). a) Show that the stress distribution y = -pg(H- y) and x = xy = 0 satisfies the two-dimensional stress equilibrium equations. y [10 marks] H b) Suppose that the concrete contains a large number of randomly oriented micro-cracks. A crack which lies at an angle to the x-axis will propagate if (1)+ foo to where and T are normal and shear stress acting on the plane defined by angle o, f is the friction coefficient between faces of the crack and to is a critical shear stress that is related to the size of the micro-cracks and the fracture toughness of the concrete, and is therefore a material property (we will talk about fracture properties in Lecture 6). Assume that f 0.5. Find the orientation of the micro-crack that is most likely to propagate. [20 marks] c) For the same friction coefficient as in b) (f = 0.5) find an expression for the maximum possible height of the column as a function of its material properties and free acceleration, g. Obtain the value of the maximum possible height of concrete columns (without reinforcement) if p = 3000 kg m, To = 50 MPa and g = 9.8 m s. [10 marks]