Question

1 Approved Answer

Posted on Jul 13, 2024

32. Assuming periodic coupons. The following is a set of Treasury auction results of various maturities: Auction: 07/14/2020 07/16/2020 07/16/2020 07/16/2020 Maturity: 1-month 3-month 6-month

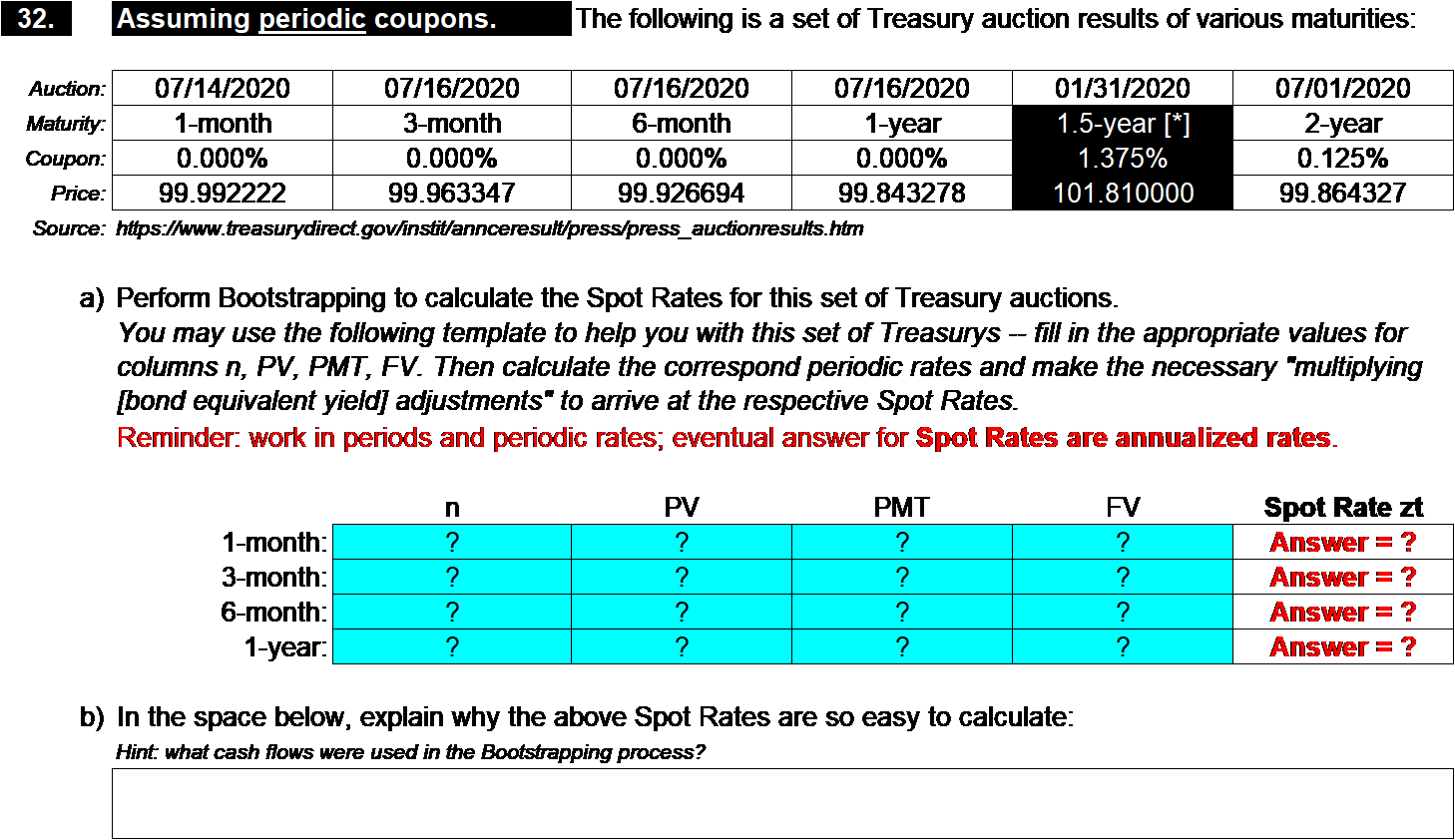
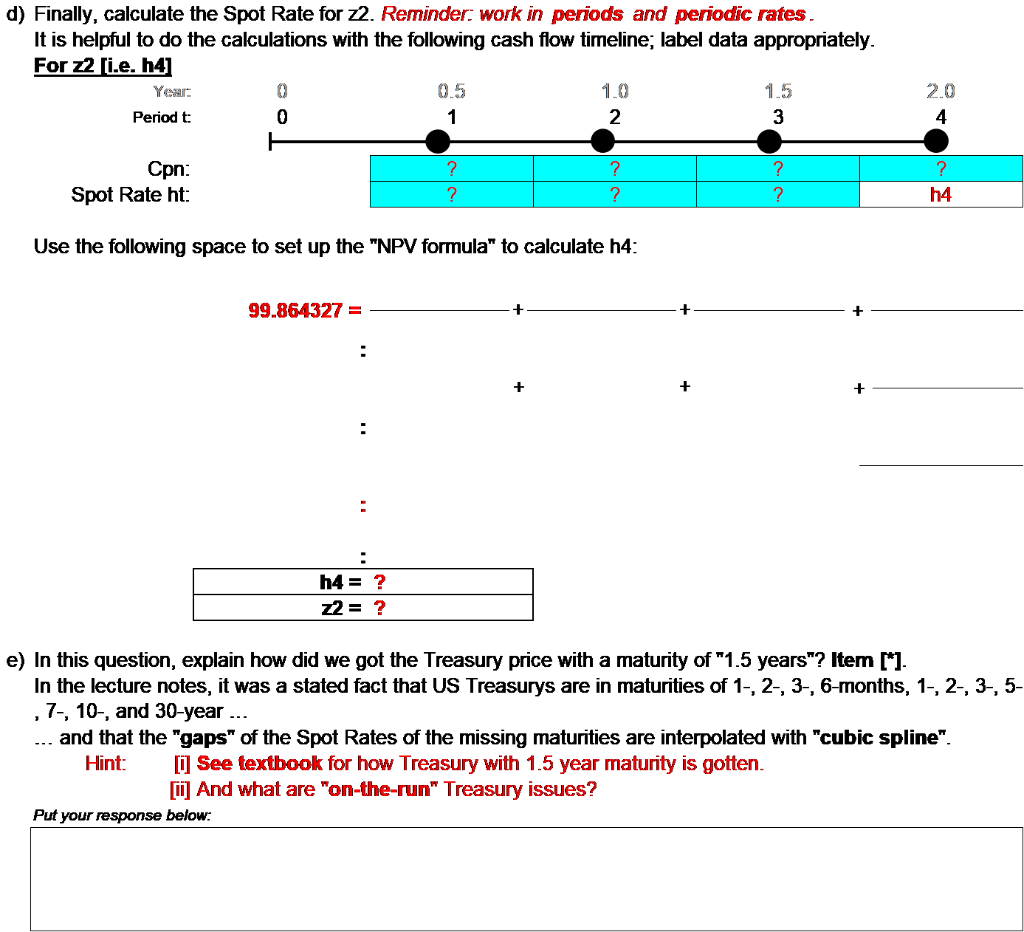
image text in transcribed

32. Assuming periodic coupons. The following is a set of Treasury auction results of various maturities: Auction: 07/14/2020 07/16/2020 07/16/2020 07/16/2020 Maturity: 1-month 3-month 6-month 1-year Coupon: 0.000% 0.000% 0.000% 0.000% Price: 99.992222 99.963347 99.926694 99.843278 Source: https://www.treasurydirect.gov/institlannceresult/press/press_auctionresults.htm 01/31/2020 1.5-year [*] 1.375% 101.810000 07/01/2020 2-year 0.125% 99.864327 a) Perform Bootstrapping to calculate the Spot Rates for this set of Treasury auctions. You may use the following template to help you with this set of Treasurys -- fill in the appropriate values for columns n, PV, PMT, FV. Then calculate the correspond periodic rates and make the necessary "multiplying [bond equivalent yield] adjustments to arrive at the respective Spot Rates. Reminder: work in periods and periodic rates; eventual answer for Spot Rates are annualized rates. PV ? PMT ? ? FV ? 1-month: 3-month: 6-month: 1-year: ? ? ? ? ? ? ? ? ? ? Spot Rate zt Answer = ? Answer = ? Answer = ? Answer = ? ? b) In the space below, explain why the above Spot Rates are so easy to calculate: Hint: what cash flows were used in the Bootstrapping process? c) Continuing from the above bootstrapping process, calculate the Spot Rate for z1.5. Reminder: work in periods and periodic rates. It is helpful to do the calculations with the following cash flow timeline; label data appropriately. For 21.5 i.e. h3] 1.0 Prd: 0 1 2 H Coupons: Con Cpn Cpn Spot Rates: h1 h2 h3 1.5 3 2 21.5 = 2 Cpn1 (1 + 1)^1 + Cpn2 (1 + h2)^2 + Cpn3 + Face (1 + h3)^3 Price Use the following space to set up the "NPV formula" to calculate h3: 101.810000 = . : . h3 = ? z1.5 = ? d) Finally, calculate the Spot Rate for 22. Reminder: work in periods and periodic rates. It is helpful to do the calculations with the following cash flow timeline; label data appropriately. For 22 (i.e. h4] 0 0.5 1.0 1.5 0 1 2 3 Year: 20 4 Period t: Cpn: Spot Rate ht: ? ? ? h4 ? Use the following space to set up the "NPV formula" to calculate h4: 99.864327 = + + + + + : : h4 = ? Z2 = ? e) In this question, explain how did we got the Treasury price with a maturity of "1.5 years"? Item (*). In the lecture notes, it was a stated fact that US Treasurys are in maturities of 1-, 2-, 3-, 6-months, 1-, 2-, 3-, 5- ,7-, 10-, and 30-year ... and that the "gaps" of the Spot Rates of the missing maturities are interpolated with "cubic spline". Hint: i] See textbook for how Treasury with 1.5 year maturity is gotten. [] And what are "on-the-run" Treasury issues? Put your response below: 32. Assuming periodic coupons. The following is a set of Treasury auction results of various maturities: Auction: 07/14/2020 07/16/2020 07/16/2020 07/16/2020 Maturity: 1-month 3-month 6-month 1-year Coupon: 0.000% 0.000% 0.000% 0.000% Price: 99.992222 99.963347 99.926694 99.843278 Source: https://www.treasurydirect.gov/institlannceresult/press/press_auctionresults.htm 01/31/2020 1.5-year [*] 1.375% 101.810000 07/01/2020 2-year 0.125% 99.864327 a) Perform Bootstrapping to calculate the Spot Rates for this set of Treasury auctions. You may use the following template to help you with this set of Treasurys -- fill in the appropriate values for columns n, PV, PMT, FV. Then calculate the correspond periodic rates and make the necessary "multiplying [bond equivalent yield] adjustments to arrive at the respective Spot Rates. Reminder: work in periods and periodic rates; eventual answer for Spot Rates are annualized rates. PV ? PMT ? ? FV ? 1-month: 3-month: 6-month: 1-year: ? ? ? ? ? ? ? ? ? ? Spot Rate zt Answer = ? Answer = ? Answer = ? Answer = ? ? b) In the space below, explain why the above Spot Rates are so easy to calculate: Hint: what cash flows were used in the Bootstrapping process? c) Continuing from the above bootstrapping process, calculate the Spot Rate for z1.5. Reminder: work in periods and periodic rates. It is helpful to do the calculations with the following cash flow timeline; label data appropriately. For 21.5 i.e. h3] 1.0 Prd: 0 1 2 H Coupons: Con Cpn Cpn Spot Rates: h1 h2 h3 1.5 3 2 21.5 = 2 Cpn1 (1 + 1)^1 + Cpn2 (1 + h2)^2 + Cpn3 + Face (1 + h3)^3 Price Use the following space to set up the "NPV formula" to calculate h3: 101.810000 = . : . h3 = ? z1.5 = ? d) Finally, calculate the Spot Rate for 22. Reminder: work in periods and periodic rates. It is helpful to do the calculations with the following cash flow timeline; label data appropriately. For 22 (i.e. h4] 0 0.5 1.0 1.5 0 1 2 3 Year: 20 4 Period t: Cpn: Spot Rate ht: ? ? ? h4 ? Use the following space to set up the "NPV formula" to calculate h4: 99.864327 = + + + + + : : h4 = ? Z2 = ? e) In this question, explain how did we got the Treasury price with a maturity of "1.5 years"? Item (*). In the lecture notes, it was a stated fact that US Treasurys are in maturities of 1-, 2-, 3-, 6-months, 1-, 2-, 3-, 5- ,7-, 10-, and 30-year ... and that the "gaps" of the Spot Rates of the missing maturities are interpolated with "cubic spline". Hint: i] See textbook for how Treasury with 1.5 year maturity is gotten. [] And what are "on-the-run" Treasury issues? Put your response below