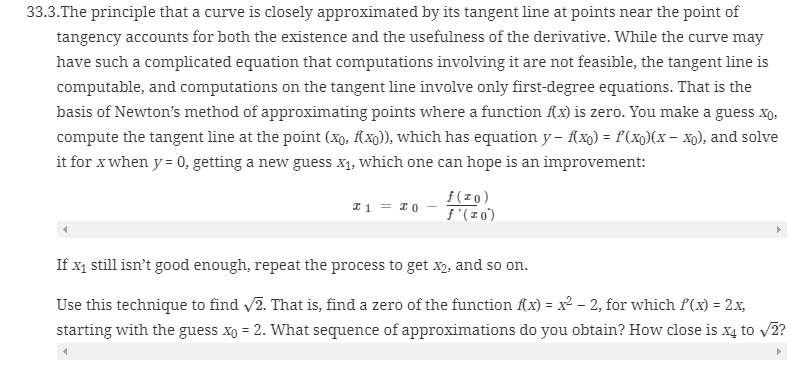
33.3. The principle that a curve is closely approximated by its tangent line at points near the point of tangency accounts for both the existence and the usefulness of the derivative. While the curve may have such a complicated equation that computations involving it are not feasible, the tangent line is computable, and computations on the tangent line involve only first-degree equations. That is the basis of Newton's method of approximating points where a function f(x) is zero. You make a guess xo, compute the tangent line at the point (x, f(x)), which has equation y f(x) = f(x)(x - xo), and solve it for x when y = 0, getting a new guess x1, which one can hope is an improvement: 21 = 20 (20) f'(20) If x still isn't good enough, repeat the process to get x2, and so on. Use this technique to find 2. That is, find a zero of the function f(x) = x - 2, for which f(x) = 2x, starting with the guess x0 = 2. What sequence of approximations do you obtain? How close is x4 to 2?