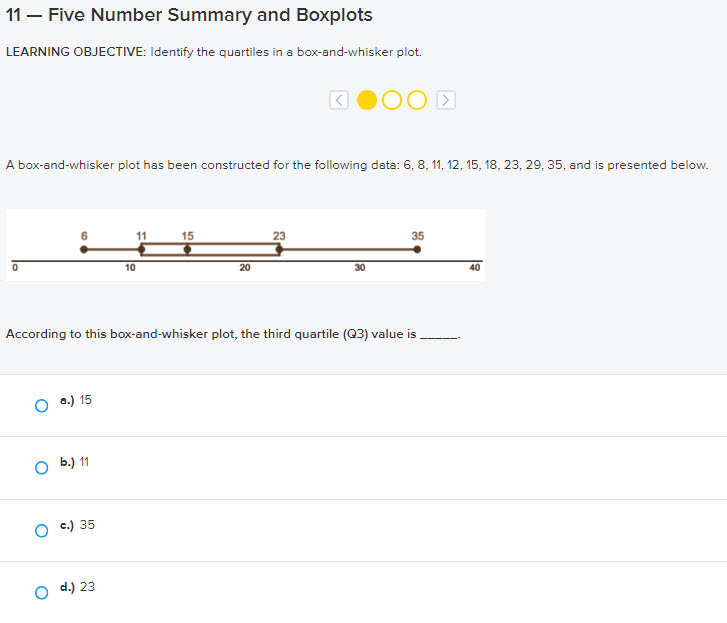
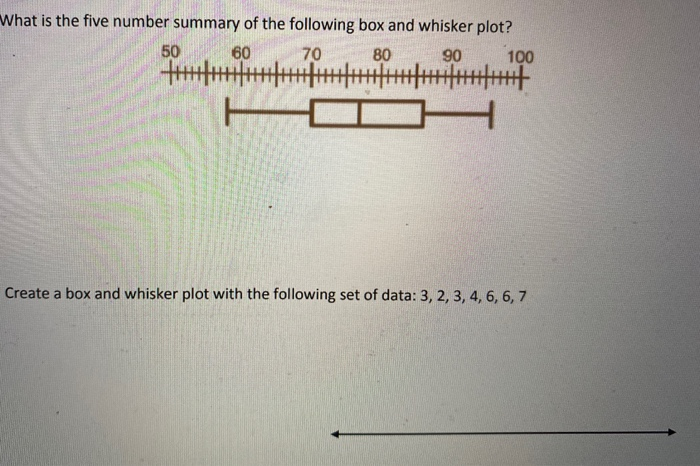
Question
36. Consider all courtroom trials with a single defendant who is charged with a felony. Suppose that you are given the following probabilities for this

36. Consider all courtroom trials with a single defendant who is charged with a felony. Suppose
that you are given the following probabilities for
this situation.
Seventy-five percent of the defendants are, in
fact, guilty. Given that the defendant is guilty,
there is a 70 percent chance the jury will convict the person. Given that the defendant is not
guilty, there is a 40 percent chance the jury will
convict the person.
For simplicity, assume that the only options
available to the jury are: to convict or to release
the defendant.
(a) What proportion of the defendants will be
convicted by the jury?
(b) Given that a defendant is convicted, what
is the probability the person is, in fact,
guilty?
(c) What is the probability that the jury will
make a correct decision?
(d) Given that the jury makes an incorrect decision, what is the probability that the decision is to release a guilty person?
37. Recall that a confidence interval is too small if
the number being estimated is larger than every
number in the confidence interval. Similarly, a
confidence interval is too large if the number
being estimated is smaller than every number
in the confidence interval.
Each of four researchers selects a random sample from the same population. Each researcher
calculates a confidence interval for the median
of the population. The intervals are below.
[24, 41], [30, 39], [20, 33], and [35, 45].
25. Bert computes a 95% confidence interval for p
and obtains the interval [0.600, 0.700]. Note:
Parts (a) and (b) are not connected: Part (b) can
be answered even if one does not know how to
do part (a).
(a) Bert's boss says, "Give me a 90% confidence interval for p." Calculate the answer
for Bert.
(b) Bert's boss says, "Give me a 95% confidence interval for p?q." Calculate the answer for Bert. (Hint: p?q = p?(1?p) =
2p ? 1. Bert's interval says, in part, that
"p is at least 0.600;" what does this tell us
about 2p ? 1?)
26. Maggie computes a 95% confidence interval for
p and obtains the interval [0.50, 0.75]. Note:
Parts (a) and (b) are not connected: Part (b) can
be answered even if one does not know how to
do part (a).
(a) Maggie's boss says, "Give me a 95% confidence interval for p
2
." Calculate the answer for Maggie. (Hint: The interval says,
in part, that "p is at most 0.75;" what does
this tell us about p
2
?)
(b) Maggie's boss says, "Give me a 95% confidence interval for p ? q." Calculate the
answer for Maggie. (Hint: p ? q = p ?
(1 ? p) = 2p ? 1. The interval says, in
part, that "p is at most 0.75;" what does
this tell us about 2p ? 1?)


Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


