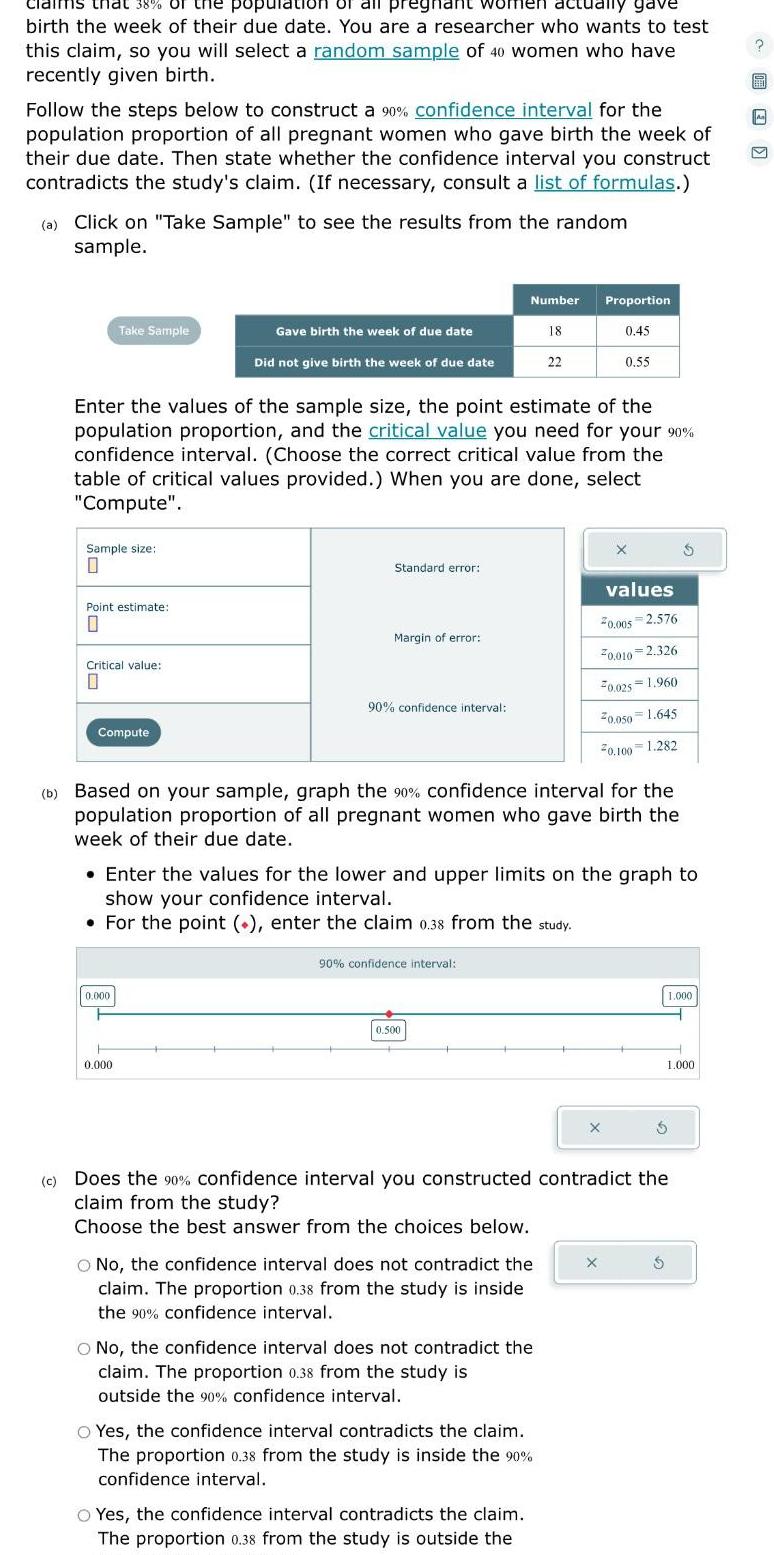
38% OT popul pregnant actual ave birth the week of their due date. You are a researcher who wants to test this claim, so you will select a random sample of 40 women who have recently given birth. Follow the steps below to construct a 90% confidence interval for the population proportion of all pregnant women who gave birth the week of their due date. Then state whether the confidence interval you construct contradicts the study's claim. (If necessary, consult a list of formulas.) (a) Click on "Take Sample" to see the results from the random sample. Number Proportion Take Sample Gave birth the week of due date 18 0.45 Did not give birth the week of due date 22 0.55 Enter the values of the sample size, the point estimate of the population proportion, and the critical value you need for your 90% confidence interval. (Choose the correct critical value from the table of critical values provided.) When you are done, select "Compute". Sample size: Point estimate: Critical value: Compute Standard error: values 20.005-2.576 Margin of error: 0.010 2.326 0.025 1.960 90% confidence interval: 0.050 1.645 0.100 1.282 (b) Based on your sample, graph the 90% confidence interval for the population proportion of all pregnant women who gave birth the week of their due date. Enter the values for the lower and upper limits on the graph to show your confidence interval. For the point (.), enter the claim 0.38 from the study. 0.000 0.000 90% confidence interval: 0.500 (c) Does the 90% confidence interval you constructed contradict the claim from the study? Choose the best answer from the choices below. No, the confidence interval does not contradict the claim. The proportion 0.38 from the study is inside the 90% confidence interval. X No, the confidence interval does not contradict the claim. The proportion 0.38 from the study is outside the 90% confidence interval. Yes, the confidence interval contradicts the claim. The proportion 0.38 from the study is inside the 90% confidence interval. Yes, the confidence interval contradicts the claim. The proportion 0.38 from the study is outside the 1.000 1.000