Question
4. [20 points] For any fixed integer k > 2, the k-PARTITION PROBLEM (abbreviated k-PP') is: given a sequence of positive integers (w, w2,... ,wn),
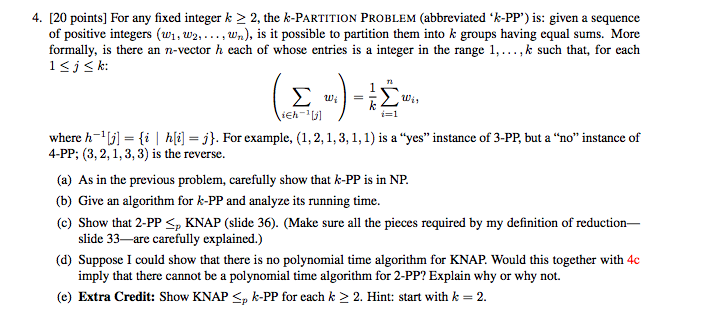
2, Hint: start with k-2. 4. [20 points] For any fixed integer k > 2, the k-PARTITION PROBLEM (abbreviated k-PP') is: given a sequence of positive integers (w, w2,... ,wn), is it possible to partition them into k groups having equal sums. More formally, is there an n-vector h each of whose entries is a integer in the range 1,..., k such that, for each 1SjSk: wis Eh where h l-i hli j. For example, (1,2,1,3,1,1) is a "yes" instance of 3-PP, but a "no" instance of 4-PP; (3, 2,1,3, 3) is the reverse. (a) As in the previous problem, carefully show that k-PP is in NP. (b) Give an algorithm for k-PP and analyze its running time (c) Show that 2-PP Sp KNAP (slide 36). (Make sure all the pieces required by my definition of reduction slide 33-are carefully explained.) (d) Suppose I could show that there is no polynomial time algorithm for KNAP. Would this together with 4c imply that there cannot be a polynomial time algorithm for 2-PP? Explain why or why not. (e) Extra Credit: Show KNAP
2, Hint: start with k-2
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


