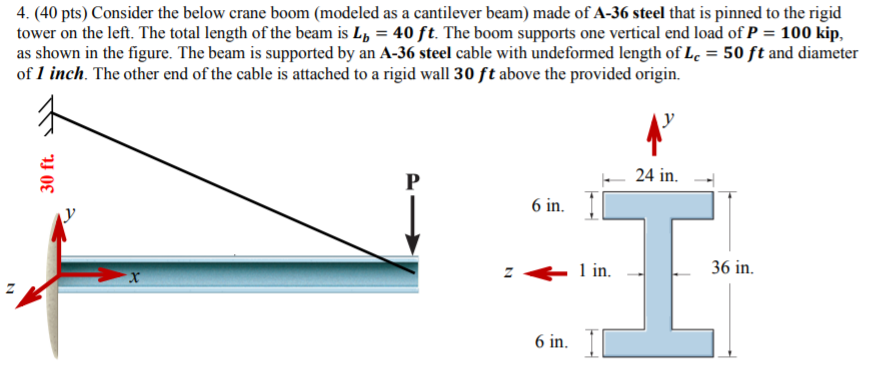
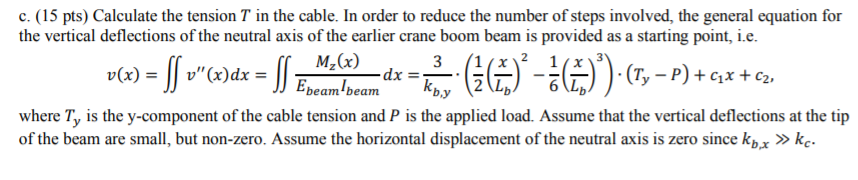
4. (40 pts) Consider the below crane boom (modeled as a cantilever beam) made of A-36 steel that is pinned to the rigid tower on the left. The total length of the beam is L = 40 ft. The boom supports one vertical end load of P = 100 kip, as shown in the figure. The beam is supported by an A-36 steel cable with undeformed length of L = 50 ft and diameter of 1 inch. The other end of the cable is attached to a rigid wall 30 ft above the provided origin. 30 ft. X P y 24 in. 6 in. 6 in. 1 in. 36 in. c. (15 pts) Calculate the tension T in the cable. In order to reduce the number of steps involved, the general equation for the vertical deflections of the neutral axis of the earlier crane boom beam is provided as a starting point, i.e. M(x) 3 v(x) = [] v" (x)dx = =SS Ebeam/beam. -dx = ( () -- ()) (Ty - P) + Gx + cz, where Ty is the y-component of the cable tension and P is the applied load. Assume that the vertical deflections at the tip of the beam are small, but non-zero. Assume the horizontal displacement of the neutral axis is zero since kb,x >> kc.