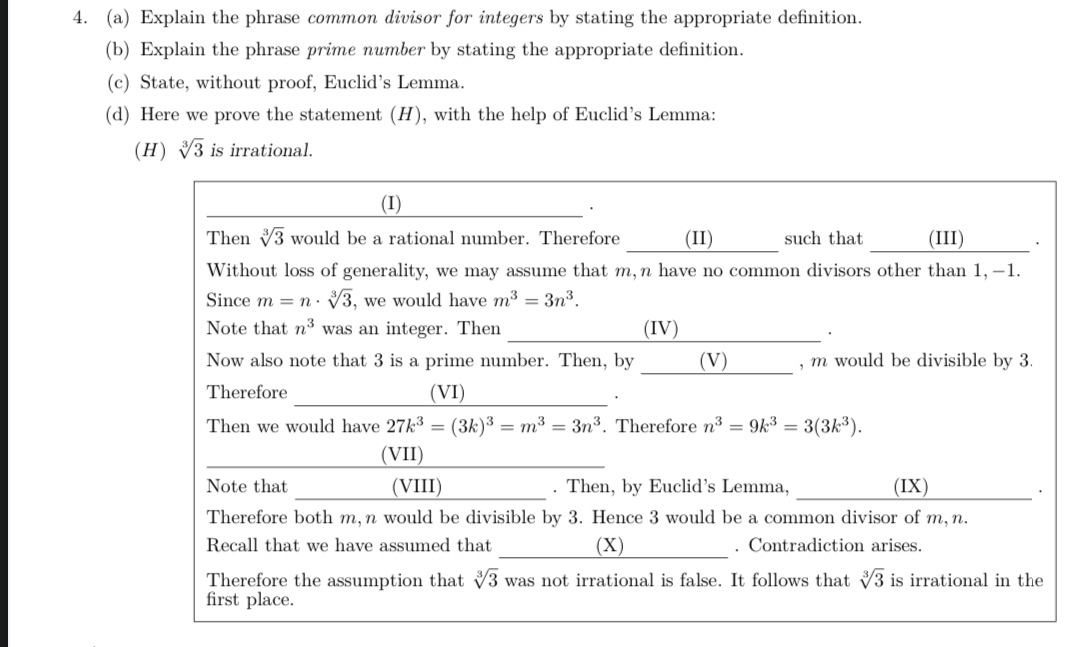
4. (a) Explain the phrase common divisor for integers by stating the appropriate definition. (b) Explain the phrase prime number by stating the appropriate definition. (c) State, without proof, Euclid's Lemma. (d) Here we prove the statement (H), with the help of Euclid's Lemma: (H) 3 is irrational. (I) such that (III) Then 3 would be a rational number. Therefore (II) Without loss of generality, we may assume that m, n have no common divisors other than 1,-1. Since m = n3, we would have m = 3n. Note that n was an integer. Then (IV) Now also note that 3 is a prime number. Then, by Therefore (VI) Then we would have 27k = (3k) =m = 3n. Therefore n = 9k = 3(3k). (VII) (V) , m would be divisible by 3. Note that (VIII) Then, by Euclid's Lemma, (IX) Therefore both m, n would be divisible by 3. Hence 3 would be a common divisor of m, n. Recall that we have assumed that (X) Contradiction arises. Therefore the assumption that 3 was not irrational is false. It follows that V3 is irrational in the first place.