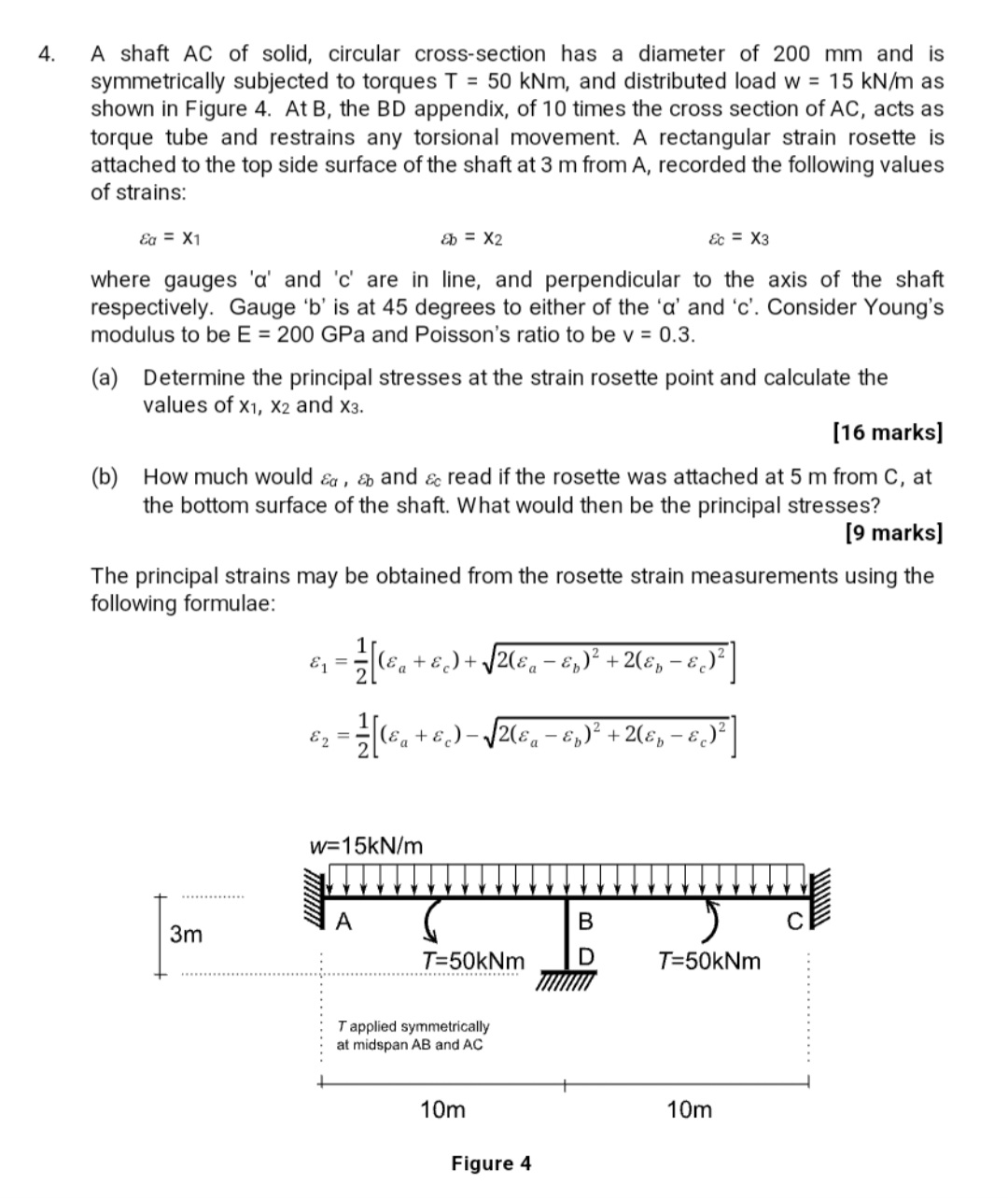
4. A shaft AC of solid, circular cross-section has a diameter of 200 mm and is symmetrically subjected to torques T = 50 kNm, and distributed load w = 15 kN/m as shown in Figure 4. At B, the BD appendix, of 10 times the cross section of AC, acts as torque tube and restrains any torsional movement. A rectangular strain rosette is attached to the top side surface of the shaft at 3 m from A, recorded the following values of strains: Ea X1 &b= X2 Ec = X3 where gauges 'a' and 'c' are in line, and perpendicular to the axis of the shaft respectively. Gauge 'b' is at 45 degrees to either of the 'a' and 'c'. Consider Young's modulus to be E = 200 GPa and Poisson's ratio to be v = 0.3. (a) Determine the principal stresses at the strain rosette point and calculate the values of X1, X2 and X3. [16 marks] (b) How much would &a, & and & read if the rosette was attached at 5 m from C, at the bottom surface of the shaft. What would then be the principal stresses? [9 marks] The principal strains may be obtained from the rosette strain measurements using the following formulae: 3m & = / [(&a + &c ) + 2(&a=&p) + 2(&p=&c E2 = / [( + & c ) - ( a - ). + 2(&p=&c) w=15kN/m A T=50kNm T applied symmetrically at midspan AB and AC 10m Figure 4 B D T=50kNm 10m C