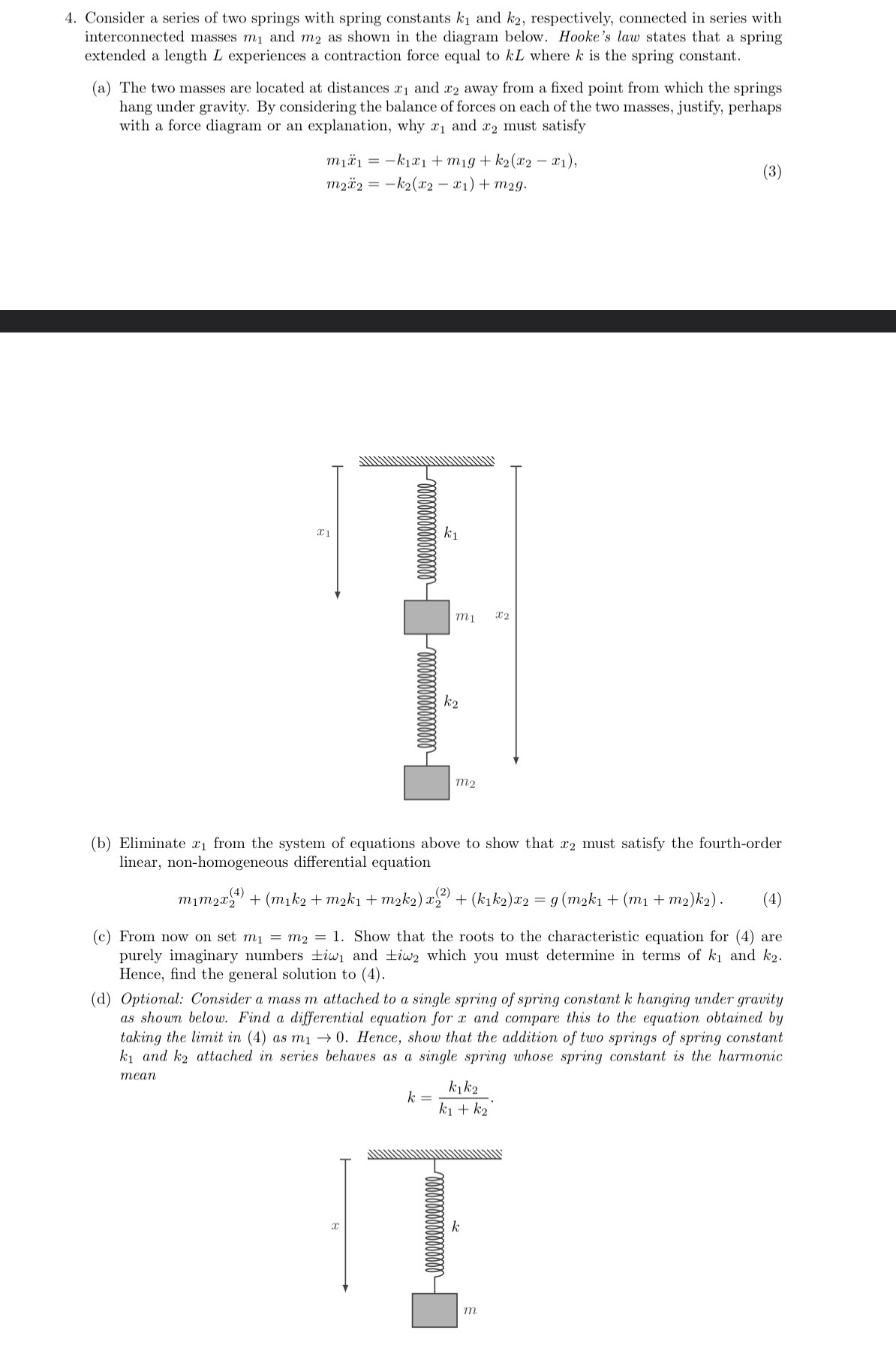
4. Consider a series of two springs with spring constants k and k2, respectively, connected in series with interconnected masses m and m2 as shown in the diagram below. Hooke's law states that a spring extended a length L experiences a contraction force equal to kL where k is the spring constant. (a) The two masses are located at distances x1 and x2 away from a fixed point from which the springs hang under gravity. By considering the balance of forces on each of the two masses, justify, perhaps with a force diagram or an explanation, why and x2 must satisfy m11 = -k1x1+m1gk2 (x2-x1), m22k2(x2-x1) +m29. (3) k 21 k2 m1 2 m2 (b) Eliminate x from the system of equations above to show that x2 must satisfy the fourth-order linear, non-homogeneous differential equation (2) m1m2x(4) + (mk2+ m2k1 + mk2) x) + (k1k2)x2 = g(m2k1 + (m + m2)k2). (4) From now on set m = m2 = 1. Show that the roots to the characteristic equation for (4) are purely imaginary numbers iw and iw2 which you must determine in terms of k and k2. Hence, find the general solution to (4). (d) Optional: Consider a mass m attached to a single spring of spring constant k hanging under gravity as shown below. Find a differential equation for x and compare this to the equation obtained by taking the limit in (4) as m 0. Hence, show that the addition of two springs of spring constant k and k2 attached in series behaves as a single spring whose spring constant is the harmonic mean k = kik2 k1+k2 M