
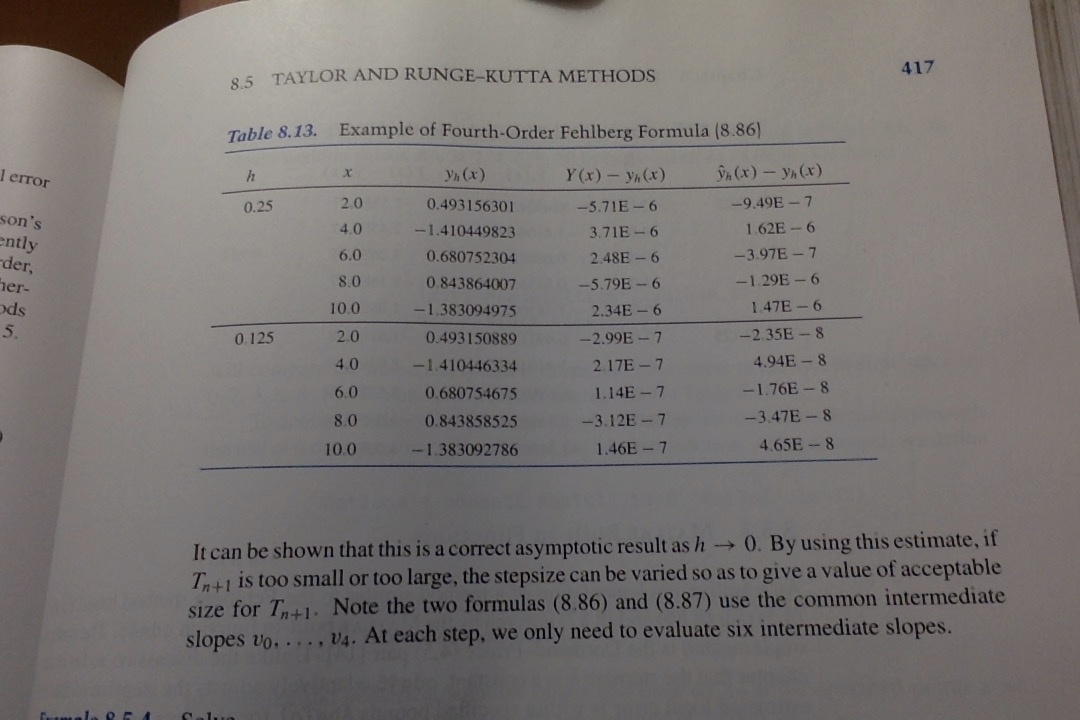
4. Consider Table 8.13 in the textbook. Using the approximations at 2 = 10.0 with h = 0.125 and h = 0.25, estimate the order of convergence of the method. Apply Richardson extrapolation to obtain a more accurate approximation of the solution at r= 10.0. Apply Richardson error estimate to estimate the error at x = 10.0 with h = 0.125. 5 TAYLOR AND RUNGE-KUTTA METHODS 417 Table 8.13. Example of Fourth-Order Fehlberg Formula (8.86) 1 error 0.25 son's ently der, 2.0 4.0 6.0 8.0 her- ods 10.0 Y() 0.493156301 -1.410449823 0.680752304 0.843864007 -1.383094975 0.493150889 -1.410446334 0.680754675 0.843858525 -1.383092786 Y(x) - Yh(x) --5.71E-6 3.71E-6 2.48E-6 -5.79E-6 2.34E-6 -2.99E-7 2.17E-7 1.14E-7 -3.12E-7 1.46E-7 n (x) - Yh(x) -9.49E-7 1.62E-6 -3.97E-7 -1.29E-6 1.47E-6 -2.35E-8 4.94E-8 -1.76E-8 -3.47E-8 4.65E-8 0.125 20 4.0 6.0 8.0 10.0 It can be shown that this is a correct asymptotic result as h0. By using this estimate, if In+ is too small or too large, the stepsize can be varied so as to give a value of acceptable size for T+1. Note the two formulas (8.86) and (8.87) use the common intermediate slopes vo...., 04. At each step, we only need to evaluate six intermediate slopes. 1 S1 4. Consider Table 8.13 in the textbook. Using the approximations at 2 = 10.0 with h = 0.125 and h = 0.25, estimate the order of convergence of the method. Apply Richardson extrapolation to obtain a more accurate approximation of the solution at r= 10.0. Apply Richardson error estimate to estimate the error at x = 10.0 with h = 0.125. 5 TAYLOR AND RUNGE-KUTTA METHODS 417 Table 8.13. Example of Fourth-Order Fehlberg Formula (8.86) 1 error 0.25 son's ently der, 2.0 4.0 6.0 8.0 her- ods 10.0 Y() 0.493156301 -1.410449823 0.680752304 0.843864007 -1.383094975 0.493150889 -1.410446334 0.680754675 0.843858525 -1.383092786 Y(x) - Yh(x) --5.71E-6 3.71E-6 2.48E-6 -5.79E-6 2.34E-6 -2.99E-7 2.17E-7 1.14E-7 -3.12E-7 1.46E-7 n (x) - Yh(x) -9.49E-7 1.62E-6 -3.97E-7 -1.29E-6 1.47E-6 -2.35E-8 4.94E-8 -1.76E-8 -3.47E-8 4.65E-8 0.125 20 4.0 6.0 8.0 10.0 It can be shown that this is a correct asymptotic result as h0. By using this estimate, if In+ is too small or too large, the stepsize can be varied so as to give a value of acceptable size for T+1. Note the two formulas (8.86) and (8.87) use the common intermediate slopes vo...., 04. At each step, we only need to evaluate six intermediate slopes. 1 S1








