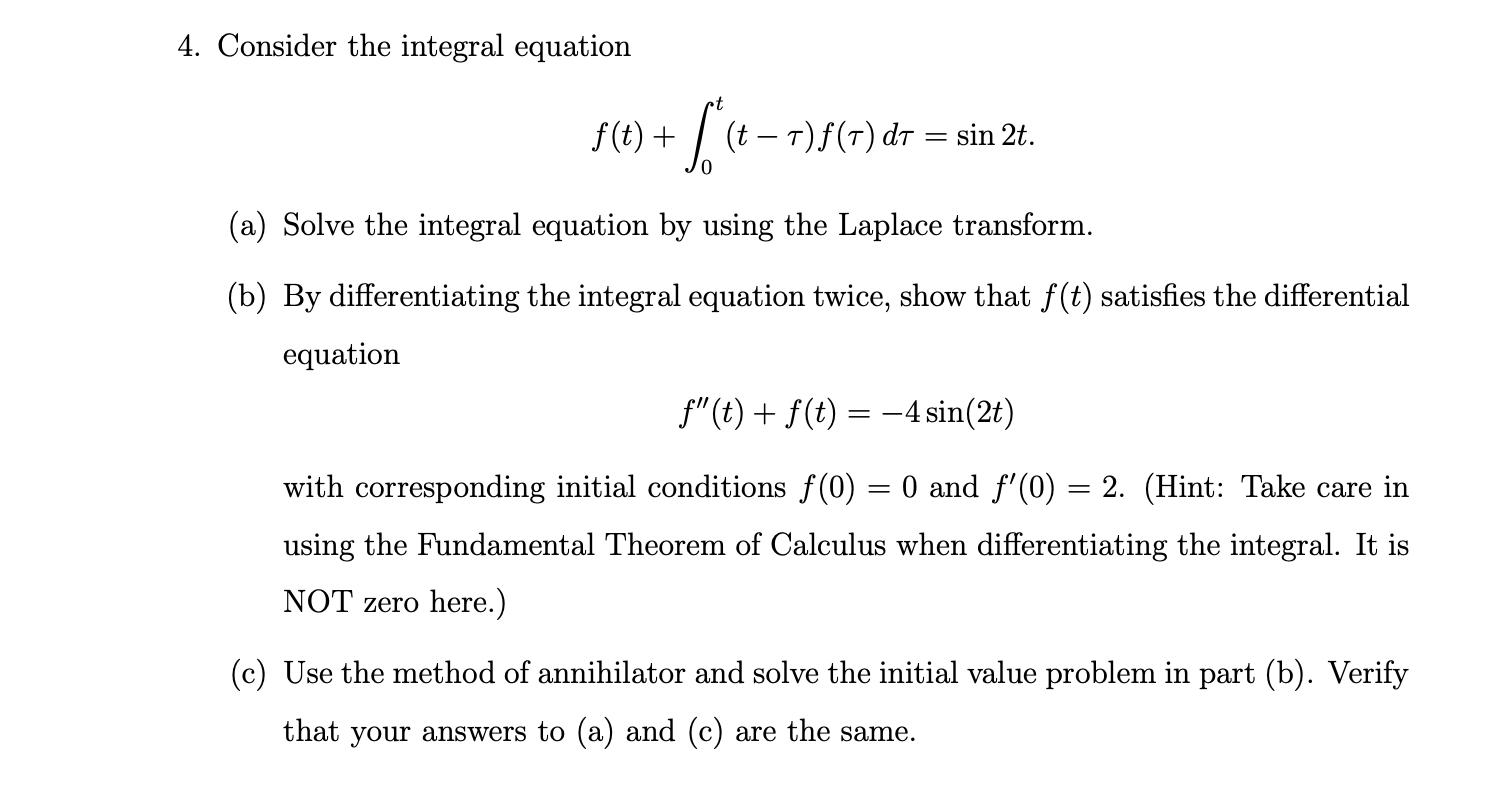
4. Consider the integral equation f(t) + L (t)f(T) dT = sin 2t. (a) Solve the integral equation by using the Laplace transform. (b) By differentiating the integral equation twice, show that f(t) satisfies the differential equation f" (t) + f (t) = 4 sin(2t) with corresponding initial conditions f(0) == 0 and f'(0) = 2. (Hint: Take care in using the Fundamental Theorem of Calculus when differentiating the integral. It is NOT zero here.) (c) Use the method of annihilator and solve the initial value problem in part (b). Verify that your answers to (a) and (c) are the same.