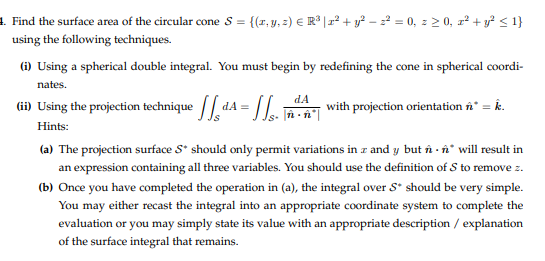
4. Find the surface area of the circular cone S = {(1, y, z) R | 1 + y z = 0, z 0, x + y 1} using the following techniques. (i) Using a spherical double integral. You must begin by redefining the cone in spherical coordi- nates. (ii) Using the projection technique dA= SS. T dA n.n Hints: with projection orientation * = k. (a) The projection surface S* should only permit variations in z and y but n. * will result in an expression containing all three variables. You should use the definition of S to remove z. (b) Once you have completed the operation in (a), the integral over S* should be very simple. You may either recast the integral into an appropriate coordinate system to complete the evaluation or you may simply state its value with an appropriate description / explanation of the surface integral that remains.