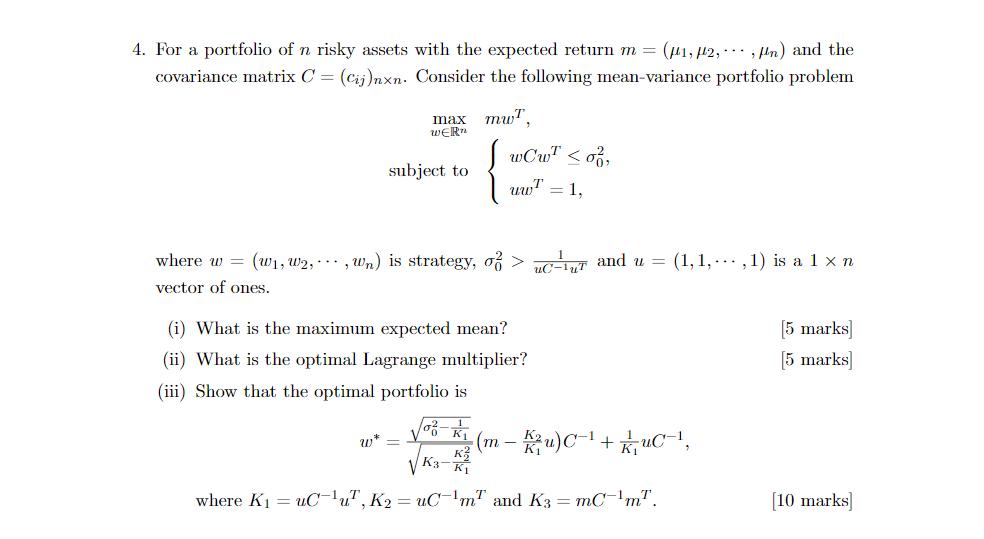
4. For a portfolio of n risky assets with the expected return m = (1, 2,,n) and the covariance matrix C = (Cij)nxn. Consider the following mean-variance portfolio problem max mwT WER wCwo, subject to uw = 1, where w = (wi, w2,, wn) is strategy, > C-1 and u = (1, 1, 1) is a 1 x n vector of ones. (i) What is the maximum expected mean? (ii) What is the optimal Lagrange multiplier? (iii) Show that the optimal portfolio is o - k (m K u) C-1 + kuc, W* K V K3- where K = uCu, K2 = uCm and K3 = mCmT [5 marks] [5 marks] [10 marks]