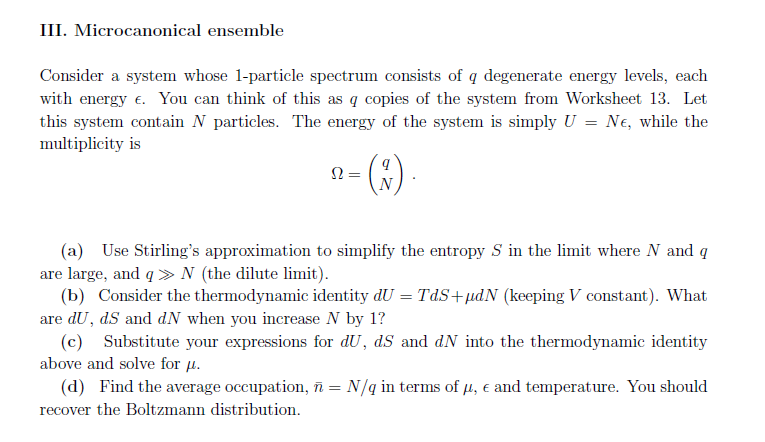
4. In the dilute limit, there are many more accessible states than particles. This implies that the average occupation of any state should be very small. We call the distribution n(, T, ) in this limit the Boltzmann distribution. In this question, will can examine it in detail in all three ensembles inctroduced in the course. I. Grand canonical ensemble (a) Consider the Bose-Einstein distribution. Fixing e and T, for what chemical potential is BE very small? (b) For the situation in the previous part, show that BE is approximately e-(E-). (c) Repeat parts (a) and (b) above for the Fermi-Dirac distribution. II. Canonical ensemble Consider a system whose 1-particle spectrum contains a level with energy (and a whole bunch other levels too). Let Z be the partition function for one particle in this system and == ZN (Z1) be the partition function for N identical particles in the dilute limit. (a) Find the chemical potential in the limit of large N (use Stirling's approximation), in terms of Z and other quantities. (b) Use your answer to part (a) to find Z in terms of the parameters , and N. (c) If the system contains just one particle, the probability of it being in our selected state with energy is e-/Z. Argue (using the fact that the system is dilute) that (the average number of particles in our selected state) is Ne-BE/Z1. (d) Substitute your result from (b) into (c) and recover the Boltzmann distribution. III. Microcanonical ensemble Consider a system whose 1-particle spectrum consists of q degenerate energy levels, each with energy . You can think of this as q copies of the system from Worksheet 13. Let this system contain N particles. The energy of the system is simply U = Ne, while the multiplicity is = (x) (a) Use Stirling's approximation to simplify the entropy S in the limit where N and q are large, and q >>> N (the dilute limit). (b) Consider the thermodynamic identity dU = TdS+dN (keeping V constant). What are du, dS and dN when you increase N by 1? (c) Substitute your expressions for dU, dS and dN into the thermodynamic identity above and solve for . (d) Find the average occupation, n = N/q in terms of , e and temperature. You should recover the Boltzmann distribution.