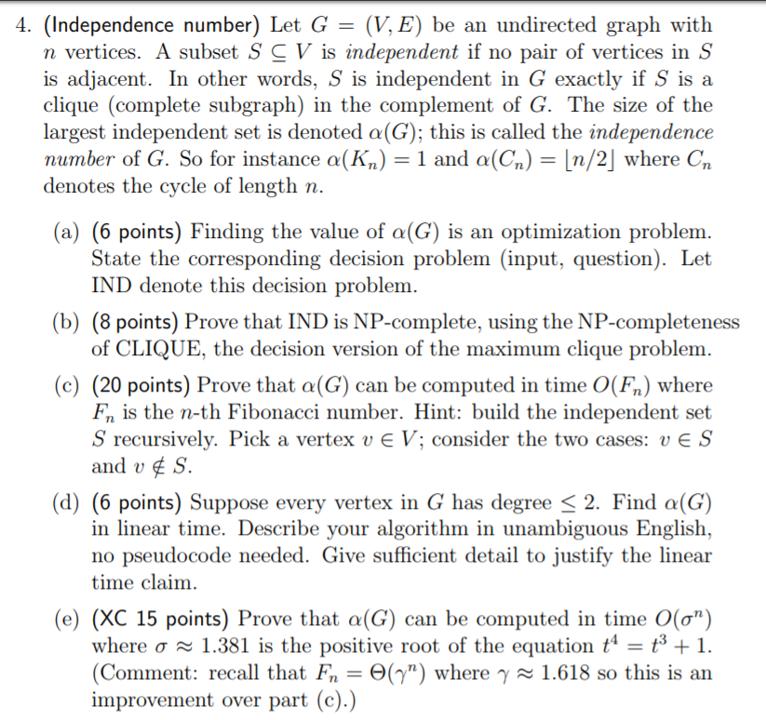
= 4. (Independence number) Let G (V,E) be an undirected graph with n vertices. A subset SCV is independent if no pair of vertices in S is adjacent. In other words, S is independent in G exactly if S is a clique (complete subgraph) in the complement of G. The size of the largest independent set is denoted a (G); this is called the independence number of G. So for instance a (Kn) = 1 and a(Cn) = [n/2] where Cn denotes the cycle of length n. (a) (6 points) Finding the value of a(G) is an optimization problem. State the corresponding decision problem (input, question). Let IND denote this decision problem. (b) (8 points) Prove that IND is NP-complete, using the NP-completeness of CLIQUE, the decision version of the maximum clique problem. (c) (20 points) Prove that a (G) can be computed in time O(F) where F is the n-th Fibonacci number. Hint: build the independent set S recursively. Pick a vertex v V; consider the two cases: v S and v S. (d) (6 points) Suppose every vertex in G has degree 2. Find a(G) in linear time. Describe your algorithm in unambiguous English, no pseudocode needed. Give sufficient detail to justify the linear time claim. (e) (XC 15 points) Prove that a(G) can be computed in time (on) where 1.381 is the positive root of the equation t = t + 1. (Comment: recall that Fn = (") where y1.618 so this is an improvement over part (c).) (").