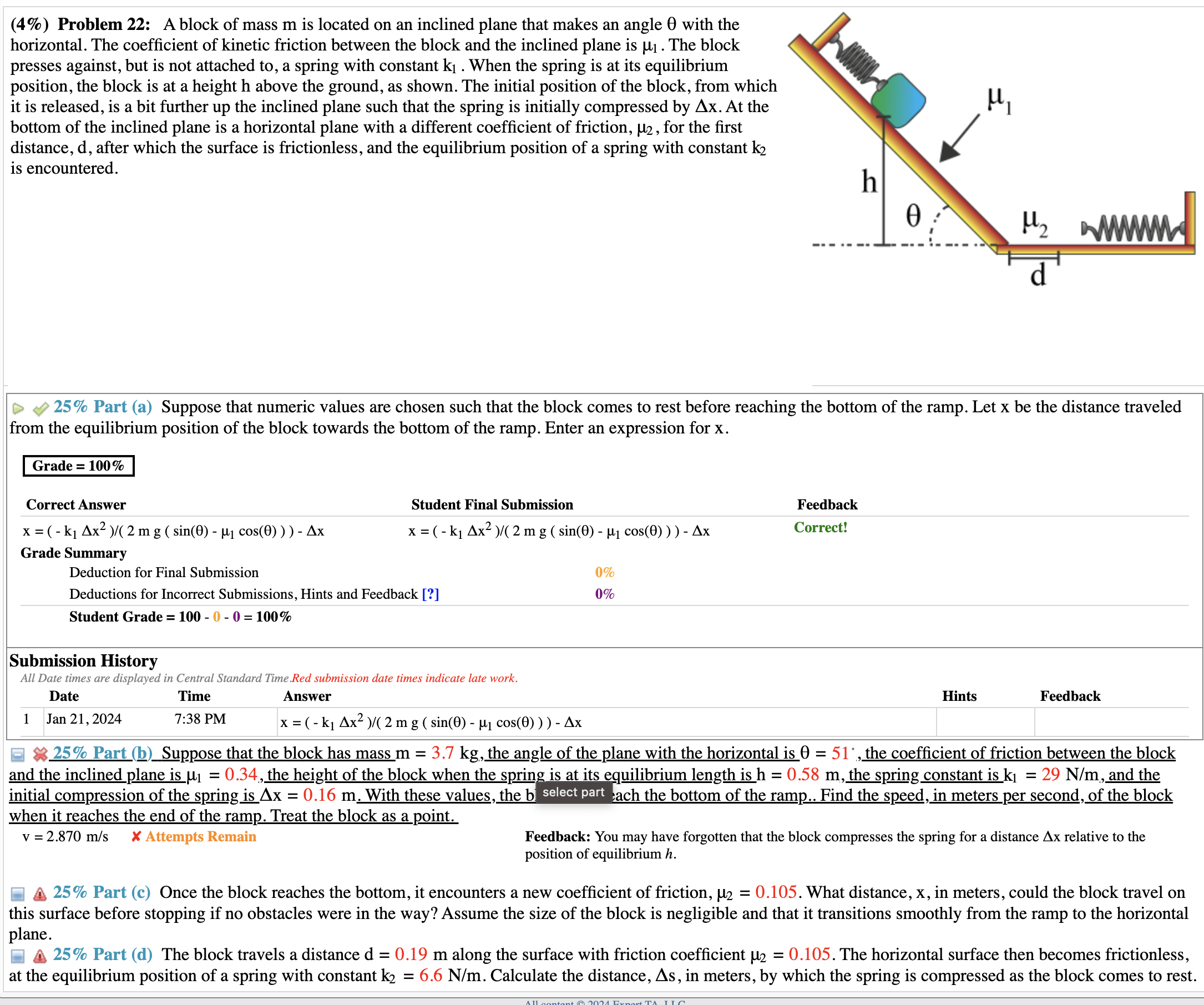
(4%) Problem 22: A block of mass m is located on an inclined plane that makes an angle 0 with the horizontal. The coefficient of kinetic friction between the block and the inclined plane is . The block presses against, but is not attached to, a spring with constant k. When the spring is at its equilibrium position, the block is at a height h above the ground, as shown. The initial position of the block, from which it is released, is a bit further up the inclined plane such that the spring is initially compressed by Ax. At the bottom of the inclined plane is a horizontal plane with a different coefficient of friction, 2, for the first distance, d, after which the surface is frictionless, and the equilibrium position of a spring with constant k is encountered. h www. d 25% Part (a) Suppose that numeric values are chosen such that the block comes to rest before reaching the bottom of the ramp. Let x be the distance traveled from the equilibrium position of the block towards the bottom of the ramp. Enter an expression for x. Grade = 100% Correct Answer Student Final Submission x = (-k Ax)/(2 mg (sin(0) - cos(0))) - Ax x = ( - k Ax)/(2 mg ( sin(0) - cos(0))) - Ax Feedback Correct! Grade Summary Deduction for Final Submission Deductions for Incorrect Submissions, Hints and Feedback [?] Student Grade = 10000 = 100% 0% 0% Submission History All Date times are displayed in Central Standard Time.Red submission date times indicate late work. Date 1 Jan 21, 2024 Time 7:38 PM Answer x = ( - k Ax)/(2 mg ( sin(0) - cos(0))) - Ax = Hints Feedback = 25% Part (b) Suppose that the block has mass m = 3.7 kg, the angle of the plane with the horizontal is 0 = 51, the coefficient of friction between the block and the inclined plane is = 0.34, the height of the block when the spring is at its equilibrium length is h 0.58 m, the spring constant is k : 29 N/m, and the initial compression of the spring is Ax = 0.16 m. With these values, the bi select part each the bottom of the ramp.. Find the speed, in meters per second, of the block when it reaches the end of the ramp. Treat the block as a point. v = 2.870 m/s * Attempts Remain Feedback: You may have forgotten that the block compresses the spring for a distance Ax relative to the position of equilibrium h. 25% Part (c) Once the block reaches the bottom, it encounters a new coefficient of friction, 2 = 0.105. What distance, x, in meters, could the block travel on this surface before stopping if no obstacles were in the way? Assume the size of the block is negligible and that it transitions smoothly from the ramp to the horizontal plane. A 25% Part (d) The block travels a distance d = 0.19 m along the surface with friction coefficient 2 at the equilibrium position of a spring with constant k = = 0.105. The horizontal surface then becomes frictionless, = : 6.6 N/m. Calculate the distance, As, in meters, by which the spring is compressed as the block comes to rest. All content 2021 Evnort TA LIC