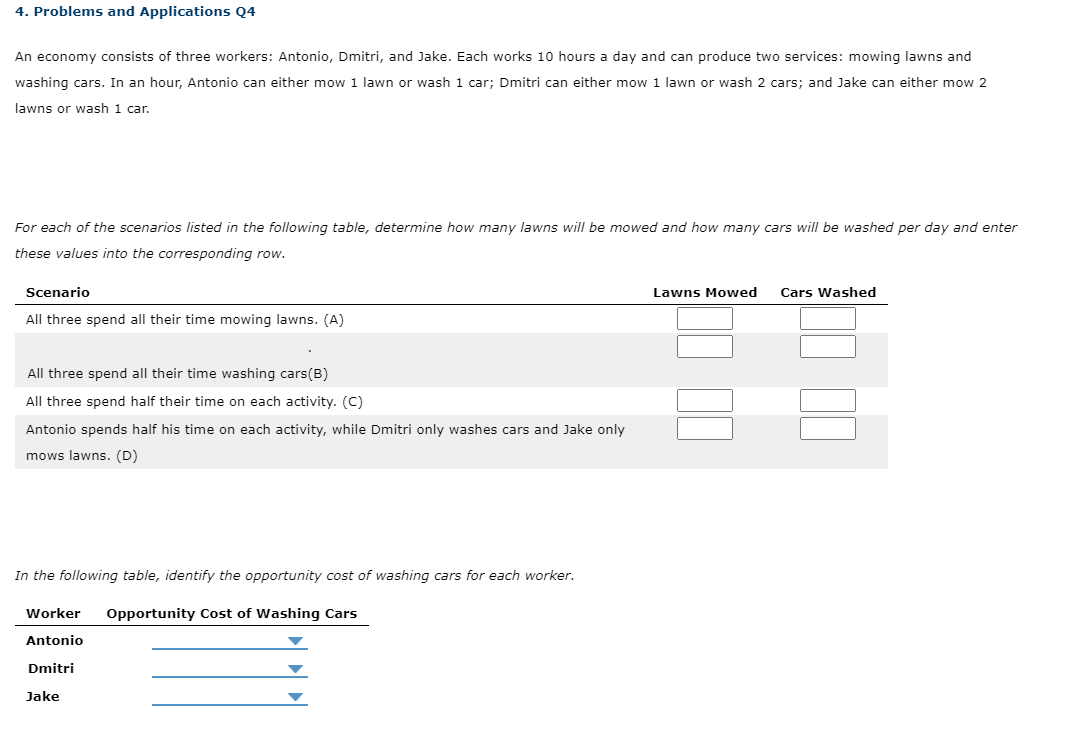
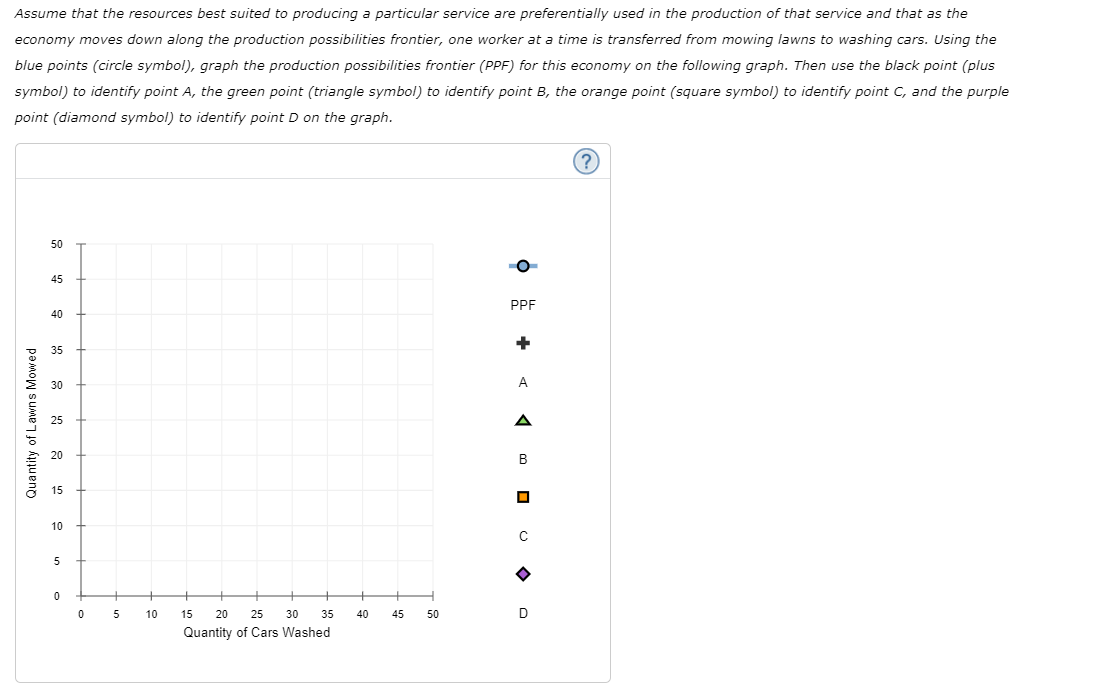
4. Problems and Applications Q4 An economy consists of three workers: Antonio, Dmitri, and Jake. Each works 10 hours a day and can produce two services: mowing lawns and washing cars. In an hour, Antonio can either mow 1 lawn or wash 1 car; Dmitri can either mow 1 lawn or wash 2 cars; and Jake can either mow 2 lawns or wash 1 car. For each of the scenarios listed in the following table, determine how many lawns will be mowed and how many cars will be washed per day and enter these values into the corresponding row. Scenario Lawns Mowed Cars Washed All three spend all their time mowing lawns. (A) All three spend all their time washing cars(B) All three spend half their time on each activity. (C) Antonio spends half his time on each activity, while Dmitri only washes cars and Jake only mows lawns. (D) In the following table, identify the opportunity cost of washing cars for each worker. Worker Opportunity Cost of Washing Cars Antonio Dmitri JakeAssume that the resources best suited to producing a particular service are preferentially used in the production of that service and that as the economy moves down along the production possibilities frontier, one worker at a time is transferred from mowing lawns to washing cars. Using the blue points (circle symbol), graph the production possibilities frontier (PPF) for this economy on the following graph. Then use the black point (plus symbol) to identify point A, the green point (triangle symbol) to identify point B, the orange point (square symbol) to identify point C, and the purple point (diamond symbol) to identify point D on the graph. 50 O A PPF 40 35 30 A 25 Quantity of Lawns Mowed A 20 15 10 5 0 5 10 15 20 25 30 35 40 45 50 D Quantity of Cars WashedTrue or False: The production possibilities frontier consists of straight-line segments, rather than being smoothly bowed outward throughout, because each worker faces a constant trade-off between mowing lawns and washing cars. O True O False Indicate whether each of the following allocations is efficient or inefficient. Allocation Efficient Inefficient A O O B O O C O O D O O