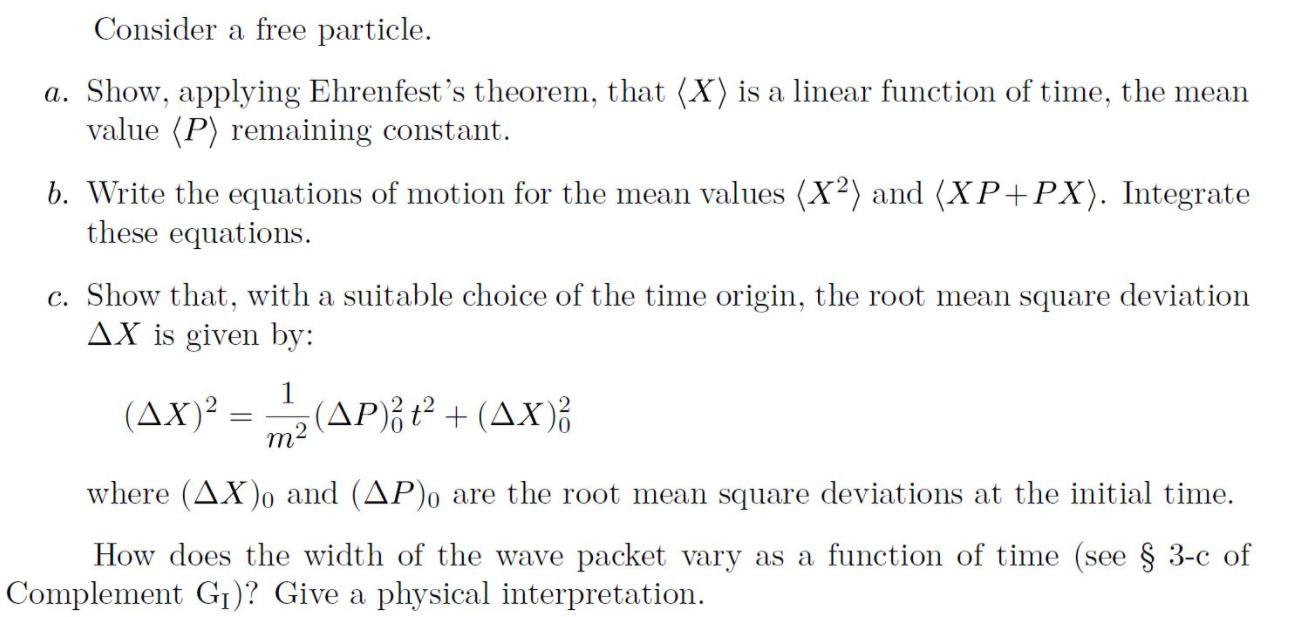
Consider a free particle. a. Show, applying Ehrenfest's theorem, that (X) is a linear function of time, the mean value (P) remaining constant. b. Write the equations of motion for the mean values (X2) and (XP+PX). Integrate these equations. c. Show that, with a suitable choice of the time origin, the root mean square deviation AX is given by: (AX). 1 (AP);t + (AX)% m2 where (AX)o and (AP)o are the root mean square deviations at the initial time. How does the width of the wave packet vary as a function of time (see 3-c of Complement G1)? Give a physical interpretation.